PDF chapter test TRY NOW
Number line:
Take a white sheet and draw a straight line. Mark the numbers left to right starting from 0.
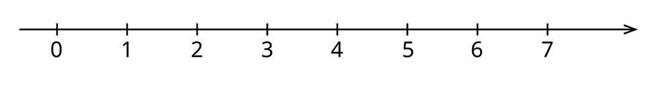
Refer to the above diagram and mark the line by numbers. The distance between any two successive numbers should be equal. That is the distance which is between 0 and 1 is the same in all the numbers. This distance is known as unit distance. Thus, all the numbers in the number line are separated by equal units.
Then what is the distance between 1 and 2? Keep thinking!...
Now we are going to see how we can do the arithmetic operation like addition, subtraction and multiplication on the number line.
Addition on the number line:
The addition of the whole can be represented using the number line figure. Now let's see how it is.
Now we are going to see the addition of 2 and 5.

We can find this using the following steps:
1. Start from 2. Since we add 5 to this number, so we have to make 5 jumps to the right side of the number line;
2. The arrow should go like 2 to 3, 3 to 4, 4 to 5, 5 to 6 and 6 to 7, as shown in the above figure. The tip of the last arrow in the fifth jump is at 7.
Therefore, we get 2 + 5 = 7.
Subtraction on the number line:
The subtraction of whole can be represented using the number line figure. Now let's see how it is.
Now we are going to see subtraction of 7 and 5.
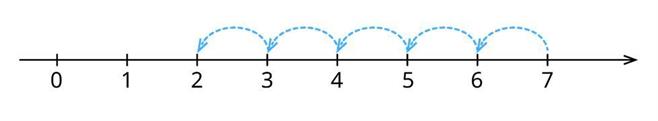
We can find this using the following steps:
1. Start from 7. Since 5 is being subtracted, so move towards the left side of the number line with 1 jump of 1 unit.
2. Make 5 such jumps. Then we reach the point 2.
Therefore, we get 7 – 5 = 2.
Multiplication on the number line:
Now let's see the multiplication of any two whole numbers on the number line.
Assume that we have to find .
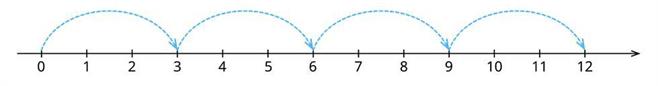
We can find this using the following steps:
1. We should start from 0, then move 3 units at a time to the right side of the number line.
2. Then make such 4 moves, after we reach 12.
Therefore we get 3 × 4 = 12.
