PDF chapter test TRY NOW
Let us discuss what linear pair of angles are.
Definition:
Linear pair is a type of angle formed by two adjacent angles, whose non-common sides are opposite rays.
In other words, the sum of the linear pair of angles is supplementary (\(180^{\circ}\)).
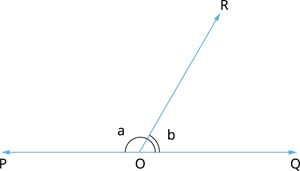
In the figure, the sum of the linear angles \(POR\) and \(ROQ\) is equal to \(180^{\circ}\).
That is, \(a + b = 180^{\circ}\).
Example:
If \(x\) and \(y\) are the measures of linear pair of angles, then find the value of \(y\) given \(x\) \(=\) \(65^{\circ}\).
Solution:
Given that, \(x\) \(=\) \(65^{\circ}\).
By the property of linear pair of angles, \(x\) \(+\) \(y\) \(=\) \(180^{\circ}\).
\(\Rightarrow 65^{\circ} + y = 180^{\circ}\)
\(\Rightarrow y = 180^{\circ} - 65^{\circ}\)
\(\Rightarrow y = 115^{\circ}\)
Some real life examples:
The following are some real-life examples where we can observe linear pair of angles.
- The ladder makes a linear pair of angles with the ground.
- The writing quill in the inked dip makes a linear pair of angle with the table.
- The knife makes a linear pair of angle with the chopping board.
Register for free to see more content