PUMPA - SMART LEARNING
எங்கள் ஆசிரியர்களுடன் 1-ஆன்-1 ஆலோசனை நேரத்தைப் பெறுங்கள். டாப்பர் ஆவதற்கு நாங்கள் பயிற்சி அளிப்போம்
Book Free DemoWe can easily represent a \(2\)-\(D\) shape on a flat surface.
But, do you think we can represent a solid shape on a flat surface?
The answer is yes and there are two ways in representing a solid shape on a flat surface, namely oblique sketch and isometric sketch.
Let us now look at each of the sketches in detail.
Oblique sketch
Oblique sketching is a pictorial representation of an object, in which the diagram is intended to depict the perspective of objects in three dimensions.
Example:
Let us try to represent a cube with dimensions of \(3\) units.
The resultant figure on a white sheet of paper would look like this.
Our aim is to recreate the figure given above on a square paper.
Let us first look at the steps involved in creating the figure.
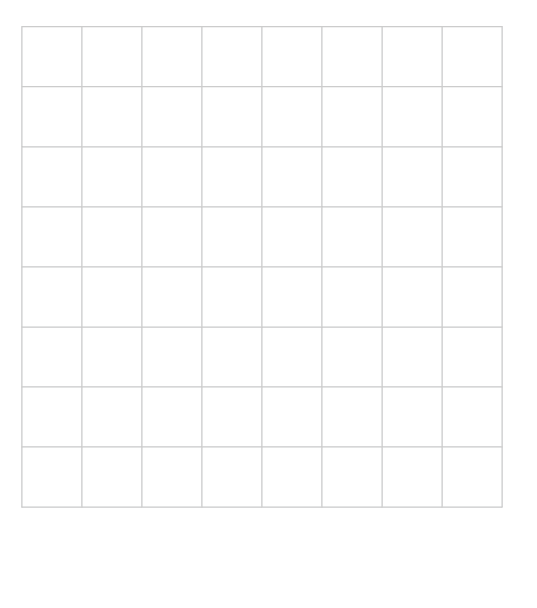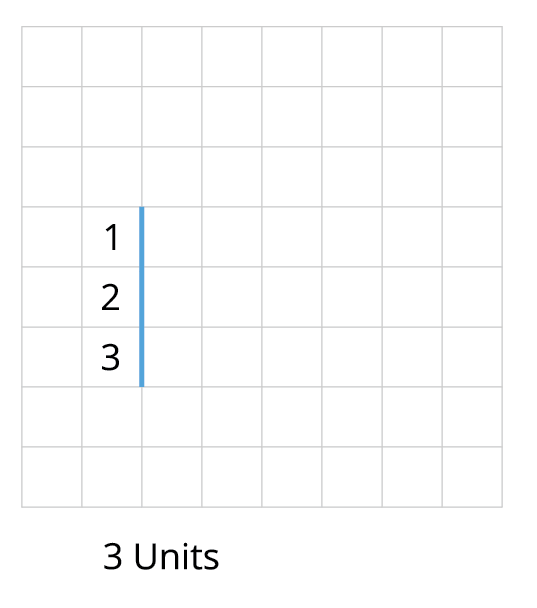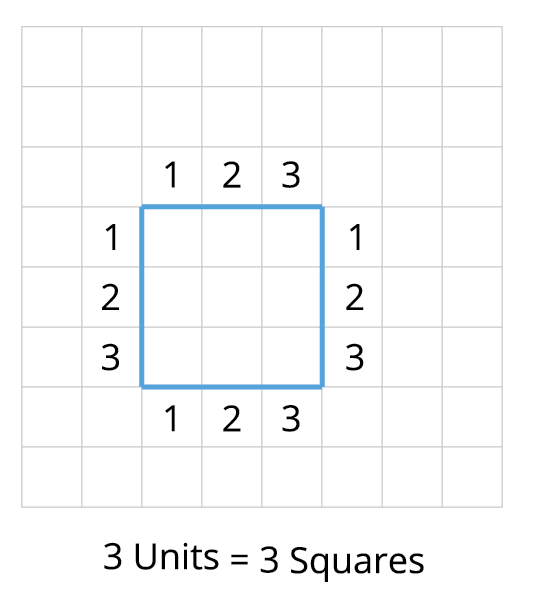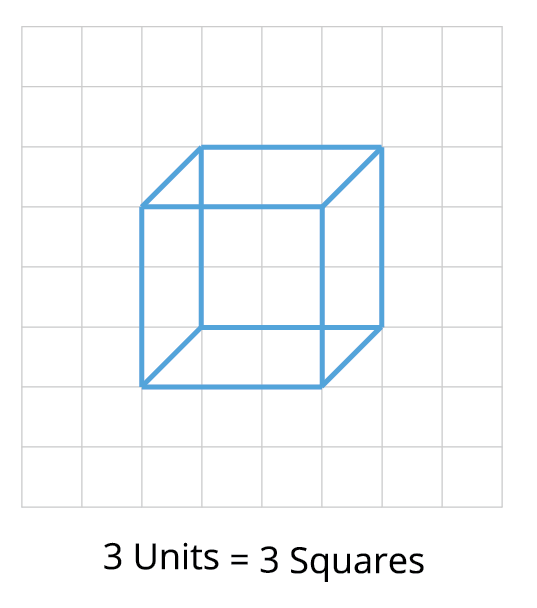
Step \(1\): Draw the front face by joining squares on the square paper, provided that the square has sides of \(3\) units.
Step \(2\): Draw another square which will form the opposite face of the square drawn previously. This opposite face could be drawn in either of the \(4\) ways given below.
In the image given above, the blue square is the front face and the pink square is the opposite face.
Step \(3\): Now join the corresponding sides of both the squares.
Step \(4\): The resultant figure forms the pictoral representation of the solid figure.
Let us find the practical way of representing the cube below:
The standard measurement is \(1\) \(\text{square}\) \(=\) \(1\) \(\text{unit}\)
Since the required cube has dimensions of \(3\) units, we should cover \(3\) squares.
Isometric sketch
Isometric sketch is similar to oblique sketching but the solid figure is represented on an isometric sheet.
What is an isometric sheet?
An isometric sheet will have dots in small equilateral triangles all over the sheet. The distance between each dot in the graph is usually considered as scale of \(1 cm\).
Example:
Let us consider creating a cuboid with \(3\) units as length, \(2\) units as breadth and \(3\) units as height on an isometric sheet.
The distance between every dot on an isometric sheet is \(1\) unit.
The practical representation looks like this:
Register for free to see more content