UPSKILL MATH PLUS
Learn Mathematics through our AI based learning portal with the support of our Academic Experts!
Learn moreWe know that a quadrilateral is made up of four sides. Any quadrilateral can be divided into two triangles with the help of a diagonal. This process of dividing a quadrilateral into triangles is called triangulation.
Let us look at the figure given below to know how a quadrilateral would look like after triangulation.
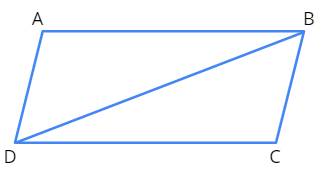
Here, \(\triangle ABD\) and \(\triangle BCD\) are formed after triangulation.
To find the area of the quadrilateral, we should know the values of \(h_1\) and \(h_2\). \(h_1\) and \(h_2\) are the perpendiculars drawn from the diagonal to the vertices. Let us look at the figure given below for a better understanding.
From the figure given above, we can come to the following inferences.
\(ABCD\) is a quadrilateral with \(d\) as the diagonal and \(h_1\) and \(h_2\) as its heights.
\(\text{Area of quadrilateral}\) \(ABCD =\) \((\text{Area of} \triangle ABD)\) \(+\) \((\text{Area of} \triangle BCD)\)
[Since the diagonal is divided into two triangles after triangulation]
\(=\) \((\frac{1}{2} \times BD \times h_1)\) \(+\) \((\frac{1}{2} \times BD \times h_2)\)
[Since,\(\text{Area of a triangle}= \frac{1}{2} \times b \times h\),where \(b\) and \(h\) are its base and height respectively]
\(=\) \(\frac{1}{2} \times BD \times (h_1 + h_2)\)
\(=\) \(\frac{1}{2} \times d \times (h_1 + h_2)\)
Therefore, \(\text{Area of a quadrilateral}\) \(=\) \(\frac{1}{2}d(h1 + h2)\) square units.
Register for free to see more content