UPSKILL MATH PLUS
Learn Mathematics through our AI based learning portal with the support of our Academic Experts!
Learn moreThere are some properties which are always true for any parallelogram.
Let's see those properties with explanation.
In a parallelogram, the following properties are true:
- the opposite sides are equal in length.
- the opposite angles are equal in measure.
- the adjacent angles are supplementary.
- the diagonals bisect each other.
Proof:
Let's prove (1) and (2).
Let ABCD be a parallelogram.
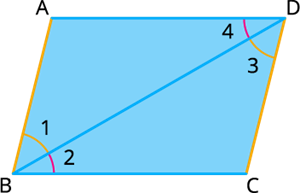
Draw a diagonal BD and denote the interior angles as ∠1, ∠2, ∠3 and ∠4.
Since ABCD is a parallelogram, AD is parallel to BC and AB is parallel to CD.
Consider the parallel lines AD and BC and take the diagonal BD as transversal.
Here ∠2 = ∠4 by alternate interior angle property [Alternate interior angles are equal in measure].
Now consider parallel lines AB and CD and take the diagonal BD as transversal.
Here ∠1 = ∠3 by alternate interior angle property [Alternate interior angles are equal in measure].
So we have ∠1 + ∠2 = ∠3+∠4. That is, ∠B = ∠D.
Consider the triangles DAB and BCD with the common side (BD = BD).
Now we have ∠2 = ∠4, ∠1 = ∠3 and the common side (BD = BD).
By ASA criterion of congruence, DAB BCD.
That is, they are congruent triangles.
Therefore, AD = BC, AC = CD and ∠A= ∠C.
Hence, in parallelogram ABCD, we have AD = BC, AC = CD, ∠A = ∠C and ∠B = ∠D.
This proves (1) and (2).
From (2), it is obvious that ∠A = ∠C and ∠B = ∠D.
Let's prove (3).
Let the measure of angles are∠A = ∠C = x and ∠B = ∠D = y.
We have the property that sum of all interior angles of a quadrilateral is 360°.
That is, ∠A + ∠B +∠C + ∠D = 360°.
Substituting the taken values in the above equation.
x + y + x + y = 360°
2x +2y = 360°
2(x + y) = 360°
x + y = 180°.
That is, we can write this as ∠A + ∠B = 180°, ∠B + ∠C = 180°, ∠C + ∠D = 180° and ∠A + ∠D = 180°.
It proves the property (3).
Let's prove (4).
Consider a parallelogram ABCD. Draw its diagonals AC and BD. Let the intersection point of the diagonals be O.
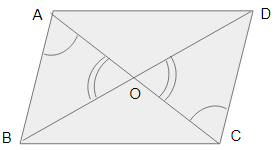
In triangle AOB and COD, we have:
AB = CD as opposite sides are equal in parallelogram.
∠AOB = ∠COD [Because vertically opposite angles are equal].
Here AB is parallel to CD, so ∠OAB = ∠DCO.
By AAS criterion of congruence, OABOCD.
This implies, OA = OC and OB = OD.
As they are equal, diagonals of a parallelogram bisect each other.