UPSKILL MATH PLUS
Learn Mathematics through our AI based learning portal with the support of our Academic Experts!
Learn moreA quadrilateral with one pair of parallel side is called trapezium.
Now we are going to define a trapezium ABCD as a quadrilateral having exactly one pair of parallel sides.
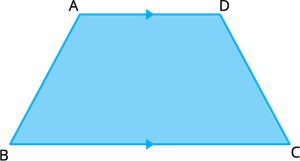
Therefore, if ABCD is a trapezium in that AD is parallel to BC(AD||BC).
Here ∠A + ∠B = 180° and ∠C + ∠D = 180°. This condition is because AD || BC and considering the line AB as transversal, the angles ∠A and ∠B are alternate interior and alternate exterior angles. Thus, they form a linear pair.
This implies, the angles ∠A and ∠B are supplementary.
Similar way, if we consider the CD as transversal, the angles ∠C and ∠D are supplementary.
Important!
Terms used in trapezium:
- The pair of parallel sides are called the bases while the non-parallel sides are called the legs of the trapezoid.
- The line segment connecting the midpoints of the non-parallel sides of a trapezoid is called the mid-segment.
- If we draw a line segment, between the two non-parallel sides, from the mid-point of both sides, the trapezium will be divided into two unequal parts.
In a trapezium, the following properties are true:
- The sum of all the four angles of the trapezium is equal to 360°.
- A trapezium has 4 unequal sides.
- A trapezium has two parallel sides and two non-parallel sides.
- The diagonals of trapezium bisect each other.
- The length of the mid-segment is equal to \frac{1}{2} the sum of the parallel bases, in a trapezium.
- Sum of adjacent angles on non-parallel sides of trapezium is 180°.
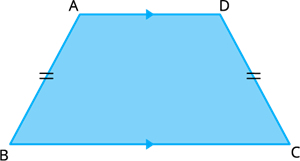
A trapezium is isosceles trapezium, if its non-parallel sides are equal.
Thus, a quadrilateral ABCD is an isosceles trapezium, if AD || BC and AB = CD.
Important!
A quadrilateral is a parallelogram if its both pairs of opposite sides are parallel.
In an isosceles trapezium, the following properties are true:
- Exactly one pair of parallel sides and one pair of congruent sides.
- Diagonals are congruent and do not bisect each other.
- Base angles are congruent, and the opposite angles are supplementary.