PDF chapter test TRY NOW
Angle Subtended by Chord at the Centre
Theorem: Equal chords of a circle subtend equal angles at the centre.
Explanation:
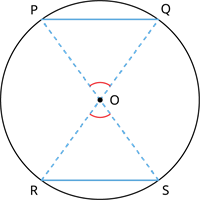
The theorem states that if the chords PQ and RS are equal, then the angle subtended by the chords respectively at the centre O are equal (i.e.) \angle POQ = \angle ROS.
Proof of the theorem:
Consider a circle with centre O and two chords PQ and RS of equal length.
Join the endpoints of the chords PQ and RS with the centre O.
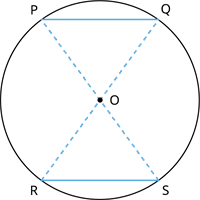
Two triangles OPQ and ORS are obtained with equal sides PQ and RS (Since the chords are equal).
The other two sides of each triangle are equal as they form the radius of the circle.
Thus, by SSS (Side-Side-Side) rule, the triangles OPQ and ORS are congruent.
Since the triangles are congruent, the corresponding parts of it are equal.
Therefore, the equal chords PQ and RS subtend equal angles at the centre.
Example:
Find the unknown angle x in the given figure where the chords PQ and RS are equal, and O is the centre of the circle.
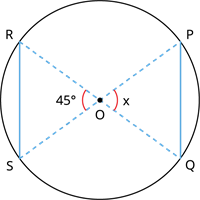
Solution:
By the above theorem, the chords PQ and RS subtend equal angle at O.
This implies that, \angle POQ = \angle ROS.
Here, \angle ROS = 45^{\circ}.
Thus, \angle POQ = 45^{\circ}.
Therefore, the unknown angle x = 45^{\circ}.
Converse of the theorem: If the angles subtended by the chords of a circle at the centre are equal, then the chords are equal.
Explanation:

The theorem states that, if PQ and RS are two chords subtending equal angle at the centre O then the chords PQ and RS equal (i.e.) PQ = RS.
Proof of the theorem:
Following the above theorem, let us find the length of the chords which subtend equal angle at the centre.
Consider a circle with centre O and two chords PQ and RS.
Join the endpoints of the chords PQ and RS with the centre O.
From the theorem, we have \angle POQ = \angle ROS.
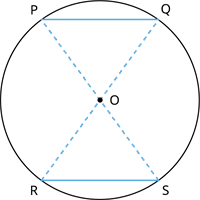
Two triangles OPQ and ORS are obtained with two sides of each triangle being equal as they form the radius of the circle.
Thus, by SAS (Side-Angle-Side) rule, the triangles OPQ and ORS are congruent.
Since the triangles are congruent, the corresponding parts of it are equal.
Therefore, the two chords PQ and RS are equal.
Example:
If two parallel chords subtend an equal angle at the centre, then prove that the chords are equal.
Solution:
Given that, the angle subtended by the two chords at the centre are equal.
According to the theorem, if the angles subtended by two chords at the centre of a circle are equal, then the chords are equal.
Therefore, the two parallel chords are equal.
Hence, proved.
