
PUMPA - SMART LEARNING
எங்கள் ஆசிரியர்களுடன் 1-ஆன்-1 ஆலோசனை நேரத்தைப் பெறுங்கள். டாப்பர் ஆவதற்கு நாங்கள் பயிற்சி அளிப்போம்
Book Free DemoNumber line:

In the above number line, the position of point P in the above line can be represented as 6 units with reference to one line (horizontal line).
A similar rule applies to the negative side of the number line as well. It is possible to represent the position of a point with reference to more than one point.
Descartes invented placing two such number lines perpendicular to each other on a plane and locating points on the plane by referring them to these lines. The perpendicular lines may be in any direction. But, when we choose these two lines to locate a point in a plane in this chapter, one line will be horizontal(First image) and the other will be vertical(Second image), as below:
Descartes invented placing two such number lines perpendicular to each other on a plane and locating points on the plane by referring them to these lines. The perpendicular lines may be in any direction. But, when we choose these two lines to locate a point in a plane in this chapter, one line will be horizontal(First image) and the other will be vertical(Second image), as below:

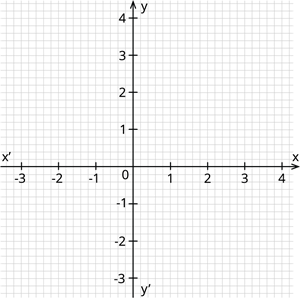
Let us combine these 2 lines in such a way they are perpendicular to each other. These two lines intersect each other at the point (0,0).
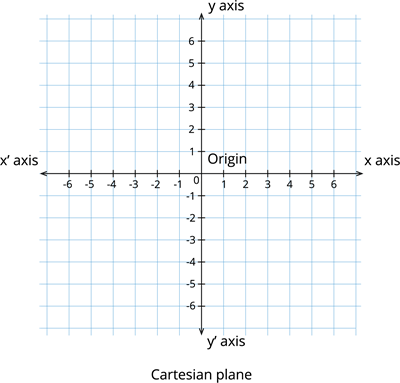
Cartesian system:
- It is a co-ordinate number system used to describe the position of a point in two dimensions by means of two perpendicular lines (x axis and y axis).
- The line X'OX is the horizontal line called the x - axis.
- The line Y'OY is the vertical line called the y - axis.
Co-ordinate axes:
The plural form of the axis is called the axes. A number line represented horizontally is the x - axis, and a number line represented vertically is the y - axis. Joining both the lines together at origin is called the xy plane or cartesian plane or co-ordinate axes.
{\color{Red} Sample}
Signs in the graphs:
- In x - axis, the point is positive along the direction OX and negative along the direction OX'.
- In y - axis, the point is positive along the direction OY and negative along the direction OY'.