PUMPA - SMART LEARNING

எங்கள் ஆசிரியர்களுடன் 1-ஆன்-1 ஆலோசனை நேரத்தைப் பெறுங்கள். டாப்பர் ஆவதற்கு நாங்கள் பயிற்சி அளிப்போம்

Book Free Demo

2. How to represent square roots of real numbers geometrically

Let us recall the concept of the square number we learned so far.

If \(a\) is a natural number, then

\sqrt{a}

\(= b\). That is, \(b² = a\) and \(b > 0\).

We are now going to extend the same definition to positive real numbers.

If \(a > 0\) be a real number, then

\sqrt{a}

\(= b\). That is, \(b² = a\) and \(b > 0\).

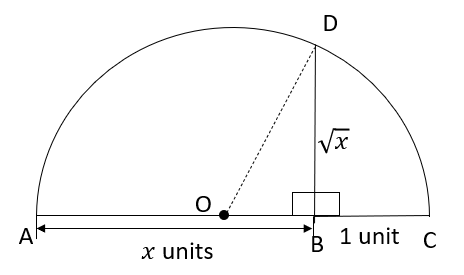
Let us learn how to find

\sqrt{x}

for any given positive real number \(x\) geometrically.

The general procedure to represent

\sqrt{x}

geometrically:

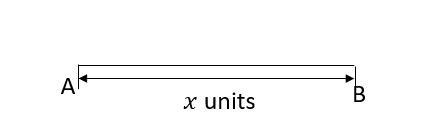
Step 1: If the given positive number is \(x\) we have to mark \(B\) so that \(AB = x\) units.

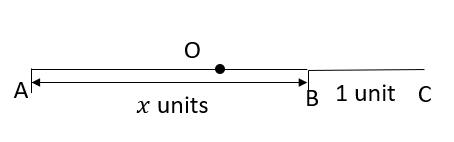
Step 2: From point \(B\), mark a distance of \(1\) unit and label the point as \(C\). That is \(BC =1\) unit.

Step 3: Now find the midpoint of \(AC\) and mark the midpoint as \(O\).

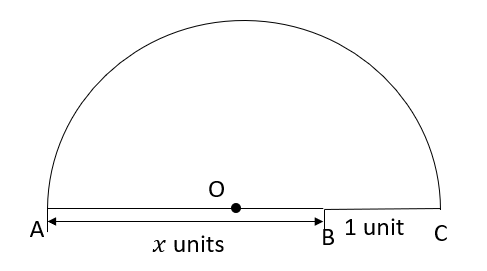
Step 4: Draw a semicircle with centre \(O\) and radius \(OC\).

Step 5: Draw a line perpendicular to \(AC\) which passes through point \(B\) and intersect the semicircle at \(D\) such that \(∠OBD = 90°\).

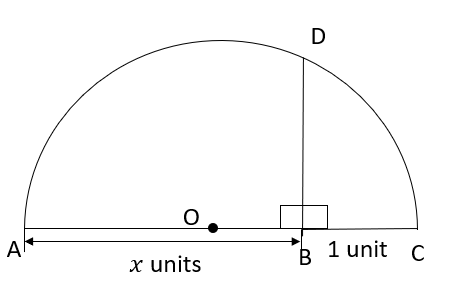
Step 6: Now measure the length of \(BD\), which is nothing but

\sqrt{x}

Let us verify the value by applying Pythagoras theorem.

Consider the right triangle \(OBD\).

\(OD²=OB²+BD²\)

We know that \(OD = OC\)(radius).

Here \(AC = AB +BC = x+1\). So \(OC = (x+1)/2 = OD\) .

Then \(OB = OC - 1 = (x+1)/2 -1 = (x-1)/2\).

\begin{array}{l} BD = \sqrt{{OD}^{2} - {OB}^{2}} \\ BD = \sqrt{{(\frac{x + 1}{2})}^{2} - {(\frac{x - 1}{2})}^{2}} \\ BD = \sqrt{\frac{x^{2} + 1 + 2 x - x^{2} - 1 + 2 x}{4}} \\ BD = \sqrt{\frac{4 x}{4}} \\ BD = \sqrt{x} \end{array}

Thus, the length of \(BD\) gives the value of

\sqrt{x}

Example:

Now we will try to find square root for the number \(4.6\)

Step 1: If the given positive number is \(x\) we have to mark \(B\) so that \(AB = 4.6\) units.

Step 2: From point \(B\), mark a distance of \(1\) unit and label the point as \(C\). That is \(BC =1\) unit.

Step 3: Now find the midpoint of \(AC = 4.6+1 = 5.6\) units and mark the midpoint as \(O\).

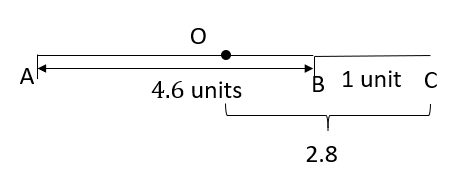
Step 4: Draw a semicircle with centre \(O\) and radius \(OC =5.6/2 = 2.8\) units.

Step 5: Draw a line perpendicular to \(AC\) which passes through point \(B\) and intersect the semicircle at \(D\) such that \(∠OBD = 90°\).

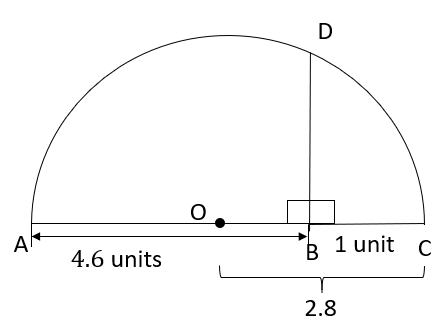
Step 6: Now measure the length of \(BD = 2.145\) which is nothing but

\sqrt{x}

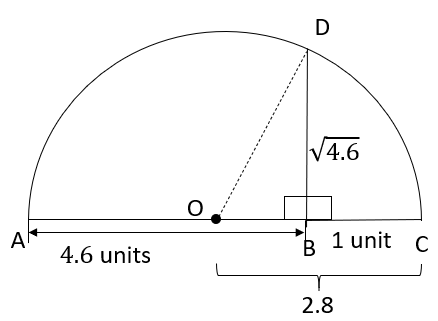
Let us verify the value by applying Pythagoras theorem.

Consider the right triangle \(OBD\).

\(OD²=OB²+BD²\)

We know that \(OD = OC = 2.8\) (radius).

Now \(OB = OC - BC = 2.8-1 = 1.8\) units

\begin{array}{l} BD = \sqrt{{OD}^{2} - {OB}^{2}} \\ BD = \sqrt{{(2.8)}^{2} - {(1.8)}^{2}} \\ BD = \sqrt{7.84 - 3.24} \\ BD = \sqrt{4.6} \\ BD = 2.145 \end{array}

Did you find an error?