PUMPA - SMART LEARNING
எங்கள் ஆசிரியர்களுடன் 1-ஆன்-1 ஆலோசனை நேரத்தைப் பெறுங்கள். டாப்பர் ஆவதற்கு நாங்கள் பயிற்சி அளிப்போம்
Book Free DemoA function is of the form \(f(x) = ax^2 + bx + c\), where \(a\), \(b\) and \(c\) are constants, and \(a \neq 0\) is called a quadratic function.
Example:
The trajectory of cables in the bridge results in a parabolic path. A parabola represents a quadratic function.
A parabola is symmetric concerning a line called the axis of symmetry. The point of intersection of the parabola and the axis of symmetry is called the vertex.
Important!
For a quadratic equation, the axis is given by \(x = \frac{-b}{2a}\) and the vertex is given by \(\left(\frac{-b}{2a}, \frac{-\Delta}{4a} \right)\) where \(\Delta = b^2 - 4ac\) is the discriminant of the quadratic equation \(ax^2 + bx + c = 0\).
A parabola usually forms a "U" shaped curve. Depending on the value of \(a\) in the general equation of the parabola \(y = ax^2\), the parabolas open upwards or downwards and vary in width.
Let us consider the simple graph \(y = x^2\).
Let us compare the above graph with graphs having the higher value of \(a\).
Here, the graph \(y = x^2\) is broader than the graph \(y = 8x^2\).
Similarly, compare graph \(y =x^2\) with graphs having lower values of \(a\).
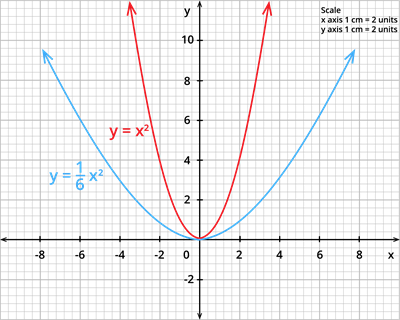
Here, the graph \(y = x^2\) is narrower than the graph \(y = \frac{1}{6}x^2\).
Important!
1. The greater the value of \(a\), the narrower is the parabola.
2. The lesser the value of \(a\), the wider is the parabola.
Reference:
https://pixabay.com/photos/golden-gate-bridge-san-francisco-388917/
Register for free to see more content