PUMPA - SMART LEARNING
எங்கள் ஆசிரியர்களுடன் 1-ஆன்-1 ஆலோசனை நேரத்தைப் பெறுங்கள். டாப்பர் ஆவதற்கு நாங்கள் பயிற்சி அளிப்போம்
Book Free DemoIn the earlier classes, we have learnt the intersection of two lines. Let us analyse what happens if a curve and a line are on a plane.
Let us discuss some situations when a circle and a line intersect.
Situation 1: The line \(AB\) does not touch the circle.
 |
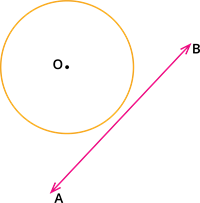There is no common point between the straight line \(AB\) and the circle.
Therefore, the number of points of intersection is zero.
Situation 2: The line \(AB\) touches the circle at one point.
 |
Here, there is one common point \(P\) between the straight line \(AB\) and the circle.
The line \(AB\) is tangent to the circle at \(P\).
Therefore, the number of points of intersection is one.
Situation 3: The line \(AB\) touches the circle at two points.
 |
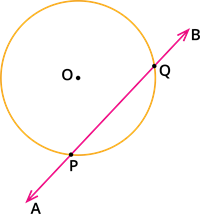Here, there are two common points \(P\) and \(Q\) between the straight line \(AB\) and the circle.
The line \(AB\) is called the secant of the circle.
Therefore, the number of points of intersection is two.
Important!
The line segment inscribed in a circle is called the chord of the circle.
 |
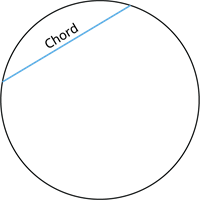The chord is a sub-section of a secant.
Register for free to see more content