Volume of sphere and hollow sphere — lesson. Mathematics State Board, Class 10.

PUMPA - SMART LEARNING

எங்கள் ஆசிரியர்களுடன் 1-ஆன்-1 ஆலோசனை நேரத்தைப் பெறுங்கள். டாப்பர் ஆவதற்கு நாங்கள் பயிற்சி அளிப்போம்

Book Free Demo

Sphere:

A sphere is a three-dimensional figure obtained by the revolution of a semicircle about its diameter as an axis.

Volume of a sphere:

Let \(r\) be the radius of a sphere.

Volume of a sphere \(=\)

\frac{4}{3} π r^{3}

cu. units

Demonstration of the volume of a sphere using right circular cones:

Let us take a sphere and two right circular cones of the same base radius and height.

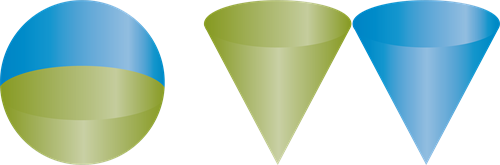
Radius of a sphere \(=\) \(r\) units

Radius of two cones \(=\) \(r\) units

Height of a sphere \(=\) Diameter \(=\) \(2r\)

Height of each cone \(=\) Height of a sphere \(=\) \(2r\)

Volume of a sphere \(=\) Volume of \(2\) cones

\(=\)

2 \times \frac{1}{3} π r^{2} h

\(=\)

2 \times \frac{1}{3} π r^{2} \times (2 r)

[Since \(h = 2r\)]

\(=\)

\frac{4}{3} π r^{3}

Volume of a sphere \(=\)

\frac{4}{3} π r^{3}

cu. units

Volume of a hollow sphere / spherical shell (volume of the material used):

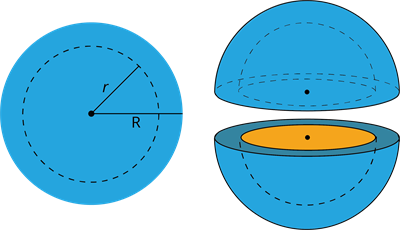
Let \(r\) be the inner radius and \(R\) be the outer radius of the hollow sphere.

Volume of a hollow sphere \(=\) Volume enclosed between the outer and inner spheres

\(=\)

\frac{4}{3} π R^{3}

\(-\)

\frac{4}{3} π r^{3}

\(=\)

\frac{4}{3} π (R^{3} - r^{3})

Volume of a hollow sphere \(=\)

\frac{4}{3} π (R^{3} - r^{3})

cu. units

Important!

The value of \(\pi\) should be taken as

\frac{22}{7}

unless its value is shared in the problem.

Did you find an error?

1. Volume of sphere and hollow sphere