The fundamental theorem of arithmetic — lesson. Mathematics State Board, Class 10.

PUMPA - SMART LEARNING

எங்கள் ஆசிரியர்களுடன் 1-ஆன்-1 ஆலோசனை நேரத்தைப் பெறுங்கள். டாப்பர் ஆவதற்கு நாங்கள் பயிற்சி அளிப்போம்

Book Free Demo

Theorem:

Every composite number can be expressed (factorised) as a product of primes, and this factorization is unique, apart from the order in which the prime factors occur.

Explanation:

Let us take the composite number

$N$ .

Decompose the number

$N$ into the product of primes.

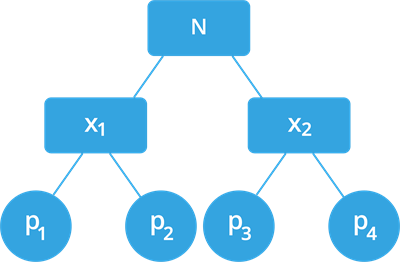
Here, the number

$N = x_1 \times x_2$ . But, both

$x_1$ and

$x_2$ are again composite numbers. So, factorise it further to obtain a prime number.

The prime factors of

$x_1 = p_1 \times p_2$ .

The prime factors of

$x_2 = p_3 \times p_4$ .

We get,

$N = p_1 \times p_2 \times p_3 \times p_4$ where

$p_1$ ,

$p_2$ ,

$p_3$ and

$p_4$ are all prime numbers.

If we have repeated primes in a product, then we can write it as a power.

In general, given a composite number

$N$ , we factorise it uniquely in the form

$N = {p_{1}}^{q_{1}} \times {p_{2}}^{q_{2}} \times {p_{3}}^{q_{3}} \times ... \times {p_{n}}^{q_{n}}$ where

$p_{1}, p_{2}, p_{3}, ... p_{n}$ are prime numbers, and

$q_{1}, q_{2}, q_{3}, ... q_{n}$ are natural numbers.

Thus, every composite number can be expressed as a product of primes apart from the order.

Example:

Consider a composite number

$26950$ .

Let us factor this number using the factor tree method.

The prime factor of

$26950$

$=$

$2 \times 5 \times 5 \times 7 \times 7 \times 11$ .

That is,

$26950 = 2 \times 5^2 \times 7^2 \times 11$ .

Here, a composite number

$26950$ is written as a product of prime numbers.

If we change the order of the prime numbers, the answer will also be the same composite number.

We can write

$26950 = 2 \times 7^2 \times 5^2 \times 11$ or

$26950 = 11 \times 7^2 \times 5^2 \times 2$ .

Thus, the prime factorization of a natural number is unique, except for the order of its factors.

Important!

Recall:

HCF

$=$ Product of the smallest power of each common prime factor in the numbers.

LCM

$=$ Product of the greatest power of each prime factor involved in the numbers.

Previous topic

Exit to the topic

Next theory

Send feedback

Did you find an error?

Send it to us!

1. The fundamental theorem of arithmetic

Theory: