UPSKILL MATH PLUS
Learn Mathematics through our AI based learning portal with the support of our Academic Experts!
Learn moreThe solutions of inequations can be represented on a number line by marking the true values of the solutions with different colours.
Let us see how the various solutions of the \(x\) can be represented graphically on the number line.
Here, we consider the solutions belong to natural numbers. That is, every value of the solution is a natural number.
Let us see how the various solutions of the \(x\) can be represented graphically on the number line.
Here, we consider the solutions belong to natural numbers. That is, every value of the solution is a natural number.
The coloured points on the number line shows the solutions of \(x\).
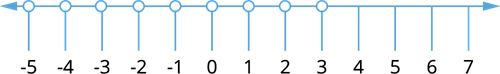
i) When \(x ≤ 4\), the solutions of \(x\) are \(4\), \(3\), \(2\), \(1\), \(0\)…... And its graph on the number as shown below.
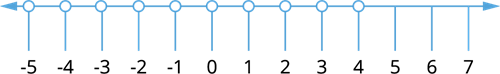
ii) When \(x ≥ 4\), the solutions of \(x\) are \(4\), \(5\), \(6\), \(7\), \(8\)…… And its graph on the number as shown below.
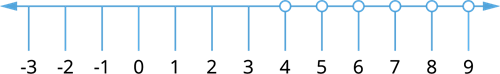
iii) Similarly, if \(x < 2\), the solutions of \(x\) are \(1\), \(0\), \(-1\), \(-2\), \(-3\)…… And its graph on the number as shown below.
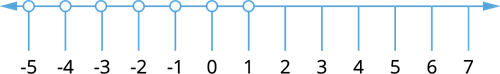
Example:
Represent the solutions of in a number line, where \(x\) is an integer.
The given expression is .
Now solve it using the inequation rules.
Step - \(1\): Subtract both sides by \(-9\).
Step - \(2\): Divide by 3 on both sides.
Since the solution belongs to integers, the solutions are 3, 2, 1, 0, −1, …. Its graph on the number line is shown below:
Register for free to see more content