Degree of expressions — lesson. Mathematics State Board, Class 7.

PUMPA - SMART LEARNING

எங்கள் ஆசிரியர்களுடன் 1-ஆன்-1 ஆலோசனை நேரத்தைப் பெறுங்கள். டாப்பர் ஆவதற்கு நாங்கள் பயிற்சி அளிப்போம்

Book Free Demo

What is meant by degree of expression?

In an expression, the terms containing the highest power of the variables is called as degree of the expression.

First, let us understand the concept of degree of variables.

Let us consider a square of side length

$x$ .

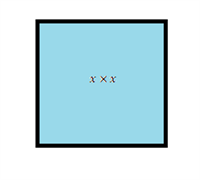
Then, the area of the square

$= x \times x$

$= x^2$

$sq. \ units$ .

Here, the expression

$x^2$ is a monomial expression, and the highest power of the expression is

$2$ which is obtained by adding the exponents.

Similarly, let us consider a rectangle whose length is

$l$ units and breadth is

$b$ units.

The area of the rectangle

$= l \times b$

$sq. \ units$

The expression is

$l^1b^1$ . Now, to find the degree of the expression, let us add the powers. Thus, we have,

$1 + 1 =2$ .

Hence, the expression

$lb$ has the highest power

$2$ , which is obtained by adding the exponents of variable factors.

Example:

1. Find the degree of the expression

$3y^2 + y +2$

Solution:

The terms in the given expression are

$3y^2$ ,

$y$ and

$2$ . Exponent of the term

$3y^2$ is

$2$ , and the exponent of the term

$y$ is

$1$ .

Here, the term

$3y^2$ has the highest degree

$2$ .

Therefore, the degree of the expression is

$2$ .

2. Find the degree of the expression

$2x^2 +3xy^4 +y+6$ .

Solution:

The terms in the given expression are

$2x^2$ ,

$3xy^4$ ,

$y$ and

$6$ .

Exponent of the term

$2x^2$ is

$2$ .

Exponent of the term

$3xy^4$ is

$1 + 4 = 5$ (Obtained by adding the variable factors).

Exponent of the term

$y$ is

$1$ .

Since, the term

$3xy^4$ has the highest degree

$5$ .

Therefore, the degree of the expression is

$5$ .

Previous theory

Exit to the topic

Next exercise

Send feedback

Did you find an error?

Send it to us!

4. Degree of expressions

Theory: