PDF chapter test TRY NOW
Let's see how to create tables and how to write linear equations using two variables for patterns.
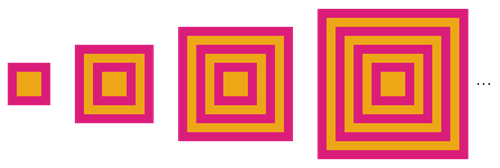
Look at the above pattern and form a table.
Let us take, \(x\) be the number of steps (or) number of columns and \(y\) be the number of square boxes within every column.
| Number of steps \((x)\) | \(1\) | \(2\) | \(3\) | \(4\) | … |
| Number of square boxes \((y)\) | \(2\) | \(4\) | \(6\) | \(8\) | … |
Now, find the relationship between the two variables \(x\) and \(y\).
For \(x = 1\), \(y = 2\)
\(y\) can be rewritten as \(y = 2 \times 1\)
As \(x = 1\), we can write \(y = 2 \times x = 2x\) - - - - - - - (I)
For \(x = 2\), \(y = 4\)
\(y\) can be rewritten as \(y = 2 \times 2\)
As \(x = 2\), we can write \(y = 2 \times x = 2x\) - - - - - - - (II)
For \(x = 3\), \(y = 6\)
\(y\) can be rewritten as \(y = 2 \times 3\)
As \(x = 3\), we can write \(y = 2 \times x = 2x\) - - - - - - - (III)
For \(x = 4\), \(y = 2\)
\(y\) can be rewritten as \(y = 2 \times 4\)
As \(x = 4\), we can write \(y = 2 \times x = 2x\) - - - - - - - (IV)
From equation (I), (II), (III) and (IV):
We can generalise the relation as \(y = 2 \times x\) or \(y = 2x\).
Register for free to see more content