PUMPA - SMART LEARNING
எங்கள் ஆசிரியர்களுடன் 1-ஆன்-1 ஆலோசனை நேரத்தைப் பெறுங்கள். டாப்பர் ஆவதற்கு நாங்கள் பயிற்சி அளிப்போம்
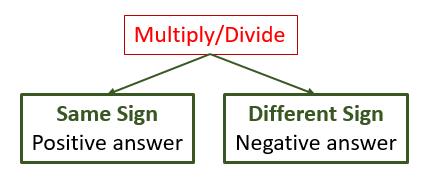
Book Free DemoWhile multiplying \(2\) integers, depending upon the sign of the two or more than two numbers, the answer can change.
1. Any one number has a negative sign:
In multiplication operation, if any one of the numbers is negative, then the result will have a negative sign \((+ve) × (-ve) = -ve\).
Example:
1.
2.
3.
2. Both numbers have a negative sign:
In multiplication operation, when both the numbers are negative, then the result will have a positive sign \((-ve)×(-ve) = +ve\). This is according to the great mathematician Euler who proved, \((-1)×(-1)=+1\).
Example:
1. \((-9) × (-10) = 90\)
2. \((-12) × (-6) = 72\)
3. \((-10) × (-10) = 100\)
4. \((-5) × (-5) = 25\)
3. Both numbers have positive sign:
In multiplication, when both the numbers are positive, the result will be a positive sign \((+ve) × (+ve) =+ve\)
Example:
1. \(12 × 10 = 120\)
2. \(10 × 6 = 60\)
3. \(10 × 5 = 50\)
4. \(8 × 6 = 48\)
Multiplication with zero:
Any integer \(×\) \(0 = 0\). Irrespective of nature and sign of integer, when an integer is multiplied by zero, the result is \(0\).
Example:
1. \(40 × 0 = 0\)
2. −682 \(× 0 =\) 0
Register for free to see more content