UPSKILL MATH PLUS
Learn Mathematics through our AI based learning portal with the support of our Academic Experts!
Learn moreTo know clearly about the perpendicular bisectors, we should understand what perpendiculars and bisectors are.
Perpendicular lines:
When two lines or line segments meet at \(90\) degrees, they are called perpendicular lines.
In the figure given above, the \(\overline{AB}\) and \(\overline{MN}\) meet each other at \(90\) degrees at \(R\).
In other words, \(\overline{AR}\) doesn't need to be equal to \(\overline{RB}\).
Bisector:
A line that divides another line into two halves is a bisector.
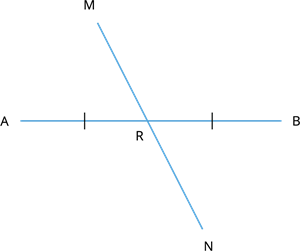
In the figure given above, \(\overline{MN}\) bisects \(\overline{AB}\) at \(R\).
Therefore, \(AR = RB\).
A perpendicular bisector:
A line or a line segment being both perpendicular and acting as a bisector is a perpendicular bisector.
In the figure given above, \(\overline{MN}\) is perpendicular to \(\overline{AB}\), and it also bisects \(\overline{AB}\).
Thus, \(\overline{MN}\) is the perpendicular bisector of \(\overline{AB}\).
Therefore, \(AR = RB\).
Register for free to see more content