UPSKILL MATH PLUS
Learn Mathematics through our AI based learning portal with the support of our Academic Experts!
Learn moreWorking rule to construct a trapezium:
Let us discuss the working rule to construct a trapezium when the measure of two sides and two angles of a trapezium are given.
Example:
Construct a trapezium \(PQRS\) in which \(\overline{PQ}\) is parallel to \(\overline{RS}\), \(PQ\) \(=\) \(8.5\) \(cm\), \(QR\) \(=\) \(6.5\) \(cm\), \(\angle PQR\) \(=\) \(75^{\circ}\) and \(\angle QPS\) \(=\) \(110^{\circ}\). Also, find its area.
Construction:
Step 1: Draw a line segment \(PQ\) \(=\) \(8.5\) \(cm\).
Step 2: With \(Q\) as centre, mark an angle \(75^{\circ}\) using a protractor and mark it as \(X\). Join \(QX\).
Step 3: With \(Q\) as centre, draw an arc of radius \(6.5\) \(cm\) intersecting \(QX\) at \(R\).
Step 4: Draw a line \(RY\) parallel to \(PQ\).
Step 5: With \(P\) as centre, mark an angle \(110^{\circ}\) using a protractor and mark it as \(Z\). Join \(PZ\) intersecting \(QY\) at \(S\).
Step 6: \(PQRS\) is the required trapezium. The measure of \(RA\) gives the height of the trapezium.
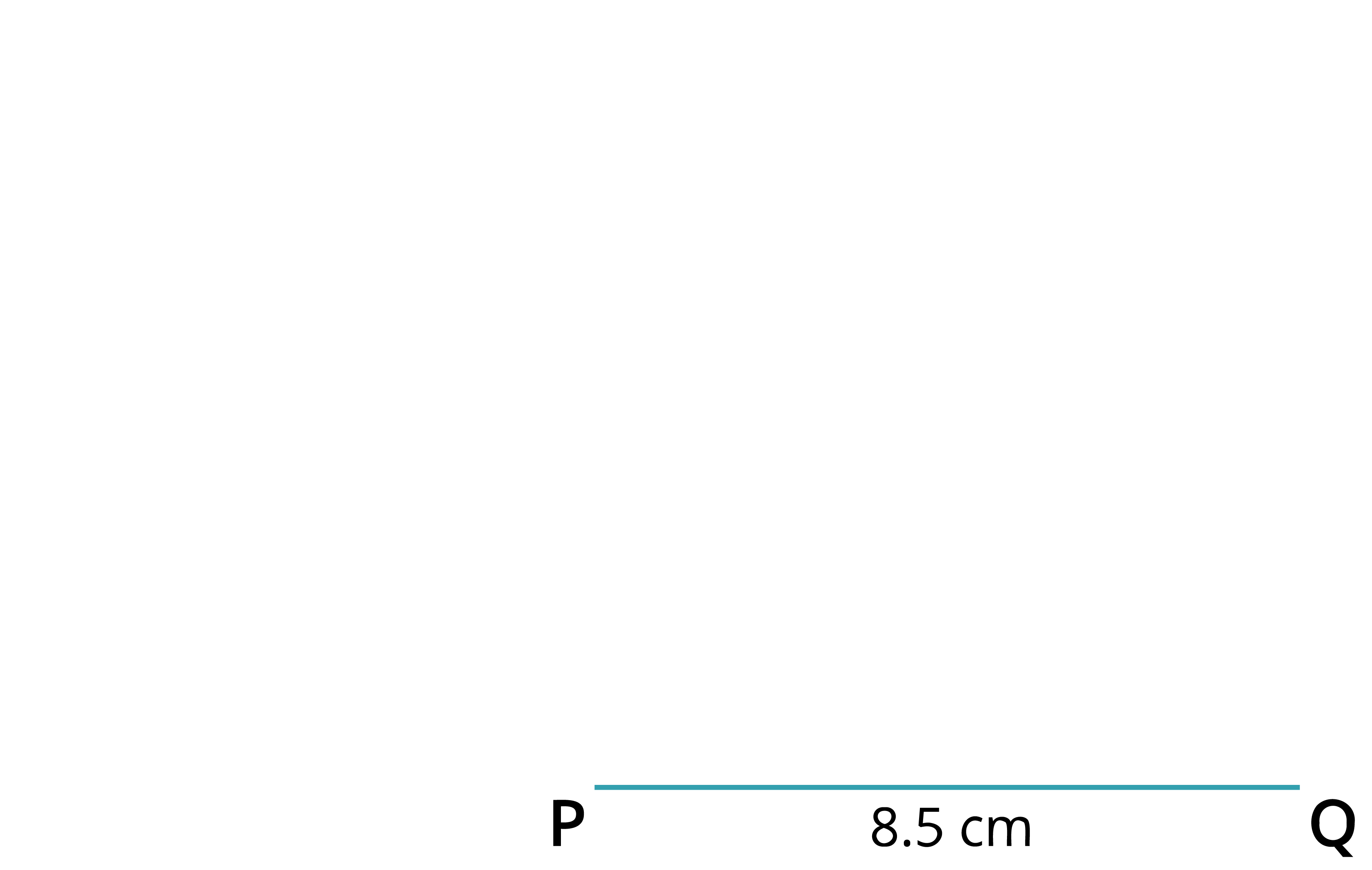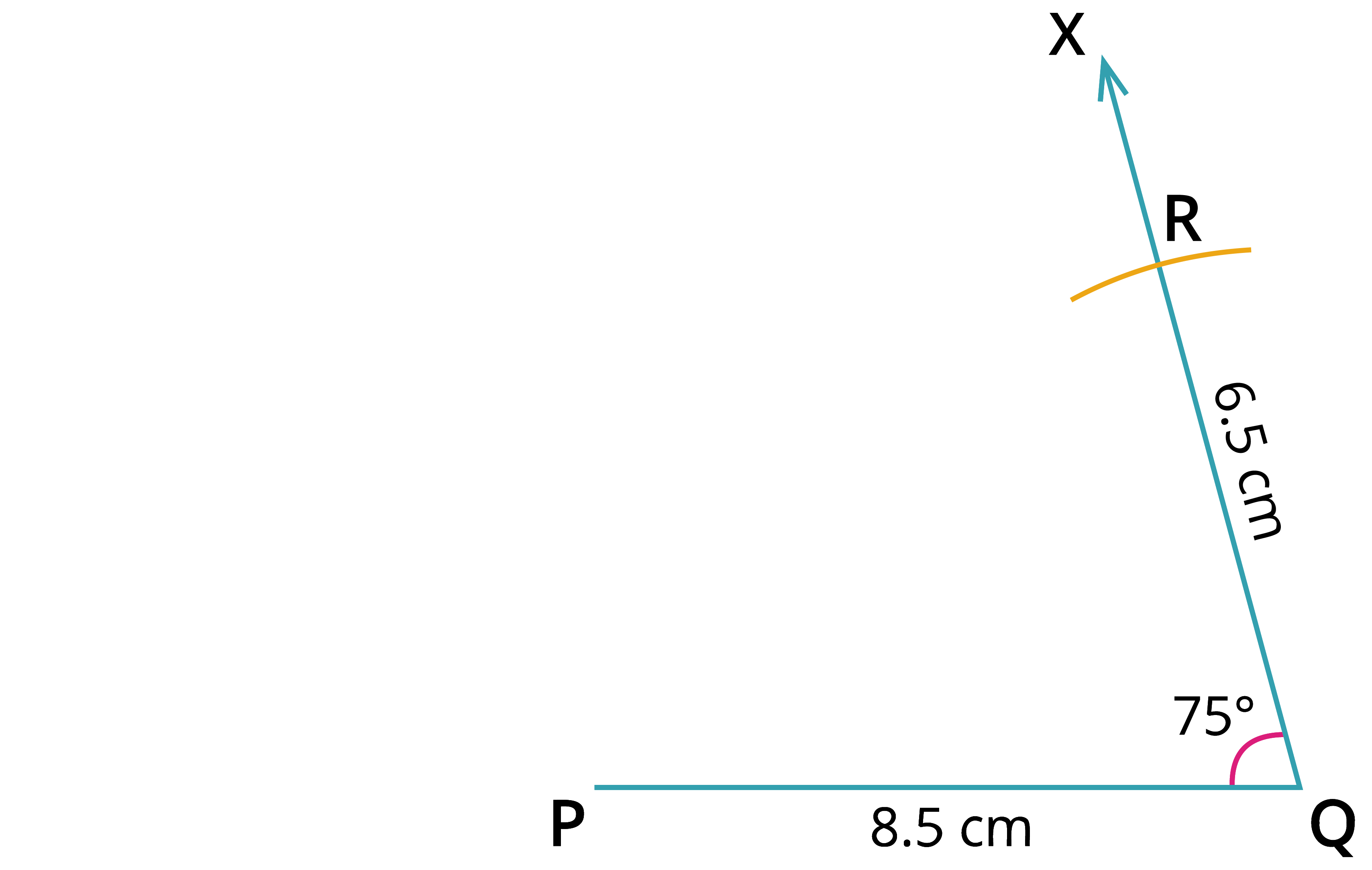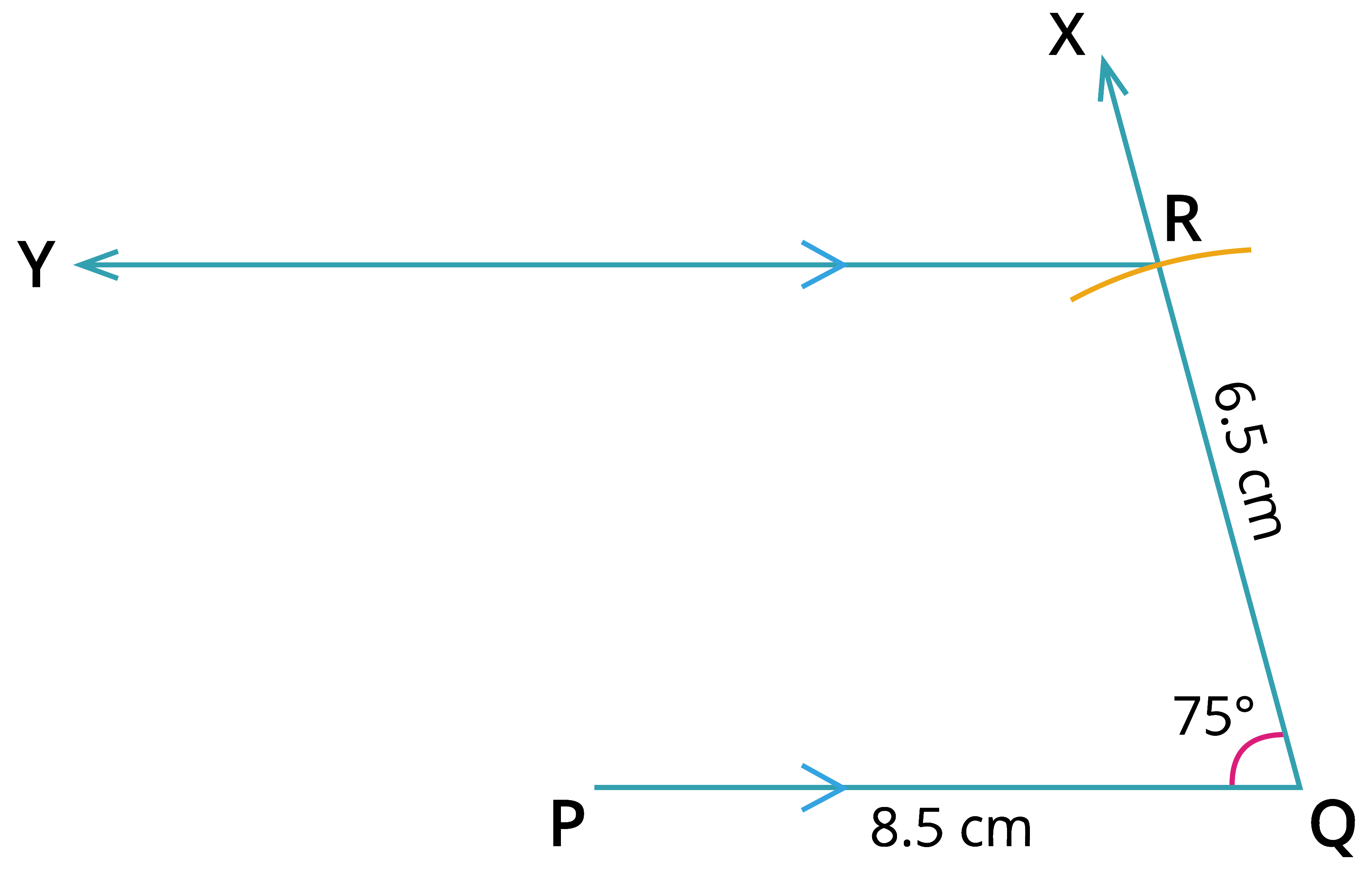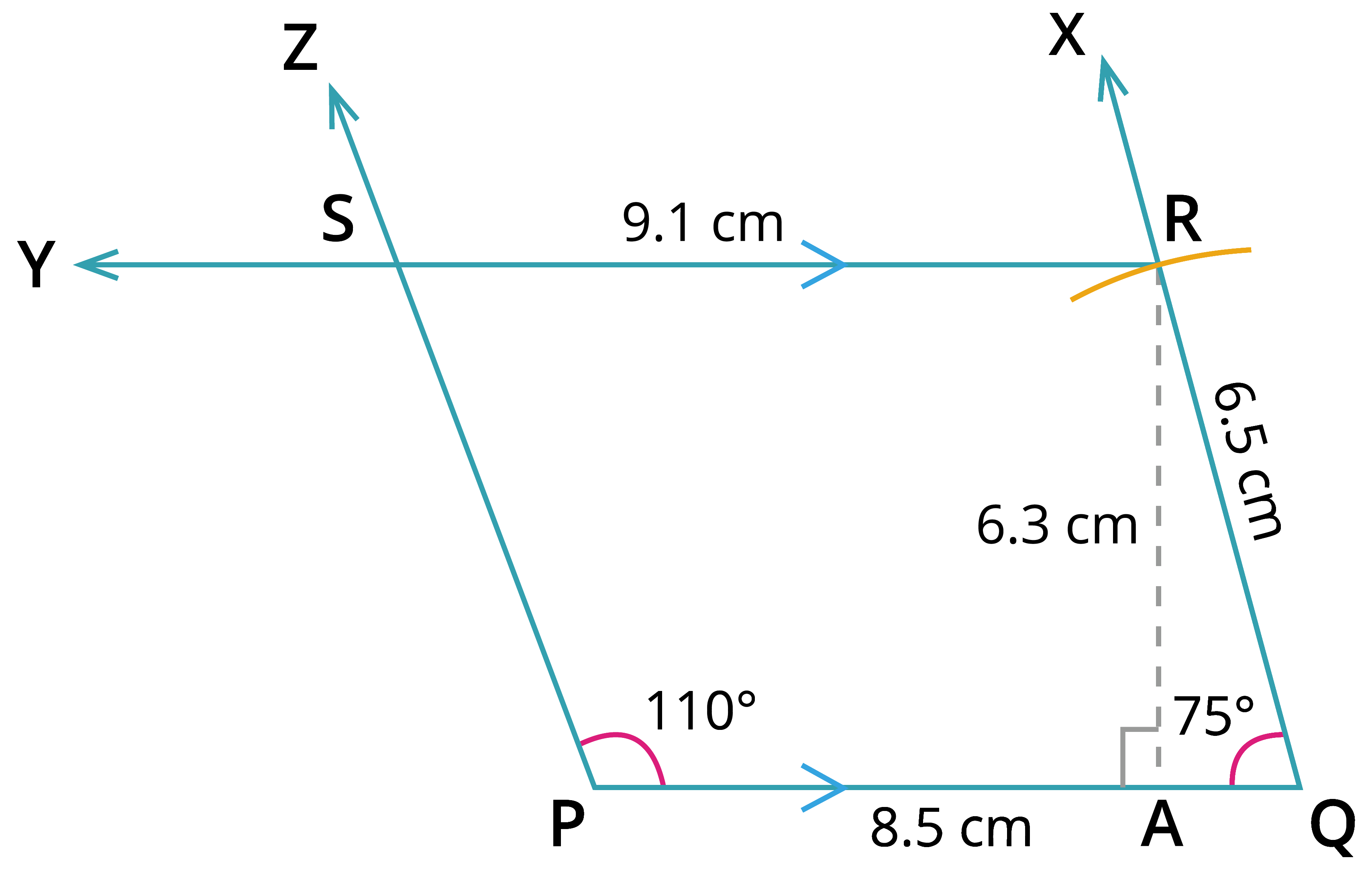
Area calculation:
Area of the trapezium \(PQRS\) \(=\) \(\frac{1}{2} \times h \times (a + b)\) square units
\(=\) \(\frac{1}{2} \times 6.3 \times (8.5 + 9.1)\)
\(=\) \(\frac{1}{2} \times 6.3 \times 17.6\)
\(=\) \(\frac{110.88}{2}\)
\(=\) \(55.44\) \(cm^2\)
Register for free to see more content