1. Pythagoras theorem

Statement:

Explanation:

 

 

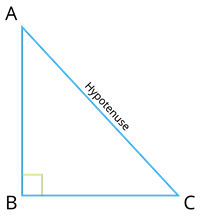
The theorem states that in the right angled triangle $ABC$, $AC^2=AB^2+BC^2$.

 

Proof of the theorem:

 

Given:

 

A triangle right angled at $B$.

 

That is $\angle ABC$ $=$ $90^{\circ}$.

 

To prove:

 

$AC^2=AB^2+BC^2$

 

Construction:

 

Construct a line from $B$ to $AC$ to intersect at $D$ such that $BD \perp AC$.

 

Proof:

 

 

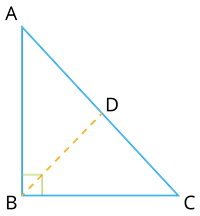
Consider the triangles $ABC$ and $BDC$.

 

The $\angle C$ is common to both the triangles.

 

Since $BD \perp AC$ we have $\angle BDC$ $=$ $90^{\circ}$.

 

Also, given that $\angle ABC$ $=$ $90^{\circ}$.

 

Thus by AA similarity (If two angles of one triangle are respectively equal to two angles of another triangle, then the two triangles are similar) it is concluded that the triangles $ABD$ and $BDC$ are similar.

 

Therefore, the ratio of the corresponding sides of the triangles are equal.

 

That is, $\frac{BC}{CD} = \frac{AC}{BC}$.

 

This implies, $BC^{2} = AC \times CD$        ……$(1)$

 

Now consider the triangles $ABC$ and $ABD$.

 

Similarly by AA criterion, the two triangles are similar.

 

So, $\frac{AB}{AD} = \frac{AC}{AB}$.

 

This implies, $AB^{2} = AC \times AD$        ……$(2)$

 

Add equations $(1)$ and $(2)$ as follows:

 

$BC^2 + AB^2$ $=$ $(AC \times CD) + (AC \times AD)$

 

$=$ $AC (CD +AD)$

 

$=$ $AC \cdot AC$

 

$=$ $AC^2$.

 

Therefore, $AC^2 = AB^2 + BC^2$.

 

Hence, the proof.