UPSKILL MATH PLUS
Learn Mathematics through our AI based learning portal with the support of our Academic Experts!
Learn moreProperties of equal chords Illustration:
Consider a circle with centre O and two equal chords PQ and RS.
Join the endpoints Q and R of the chords PQ and RS.
Draw a line OA perpendicular to RS and OB perpendicular to PQ.

Two right triangles OAR and OBQ are obtained where \angle OAR = \angle OBQ = 90^{\circ} since OA \perp RS and OB \perp PQ.
Here, OP and OQ are radius. So, they are equal.
According to the theorem, the perpendicular from the centre of the circle bisects the chord. So, RA = BQ since the chords are equal.
Therefore, by the RHS rule(In two right-angled triangles, if the length of the hypotenuse and one side of one triangle is equal to the length of the hypotenuse and corresponding side of the other triangle, then the two triangles are congruent), the triangles are congruent.
This implies that the sides OA = OB.
Based on this congruency, the following theorems are obtained.
Theorem: Equal chords of a circle are equidistant from the centre.
Explanation:

The theorem states that if the chords PQ and RS are equal, then the distance between the chords PQ and RS from the centre O are equal.
\text{Distance from }O\text{ to chord }PQ = \text {Distance from }O\text{ to chord} RS
Example:
Given, a circle with two equal and parallel chords. If one of the chords is at a distance of 5 cm from the centre, find the distance between the chords.
Solution:
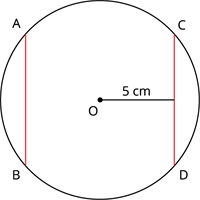
Let O be the centre of the circle and AB and CD be the equal and parallel chords.
Given, one of the chords is at a distance of 5 cm from the centre.
Let the chord CD be at a distance of 5 cm from the centre.
By the theorem, the equal chords AB and CD of a circle are equidistant from the centre.
Thus, the distance between the chord AB and the centre O is 5 cm.
Therefore, the distance between the two chords is given as follows:
= 5 cm + 5 cm
= 10 cm
Converse of Theorem: The chords of a circle which are equidistant from the centre are equal.
Explanation:

The theorem states that, if the distance between the chords PQ and RS from the centre O are equal, then the chords PQ and RS are equal (i.e.) PQ = RS.
Example:
If two chords are connected by a line segment, and the centre of the circle passes through the mid-point of the line segment, then prove that the chords are equal.
Solution:
Given that, the centre of the circle passes through the mid-point of the line segment connecting the chords.
This implies that the chords are equidistant from the centre.
Therefore by the theorem, the chords are equal.
Hence, proved.