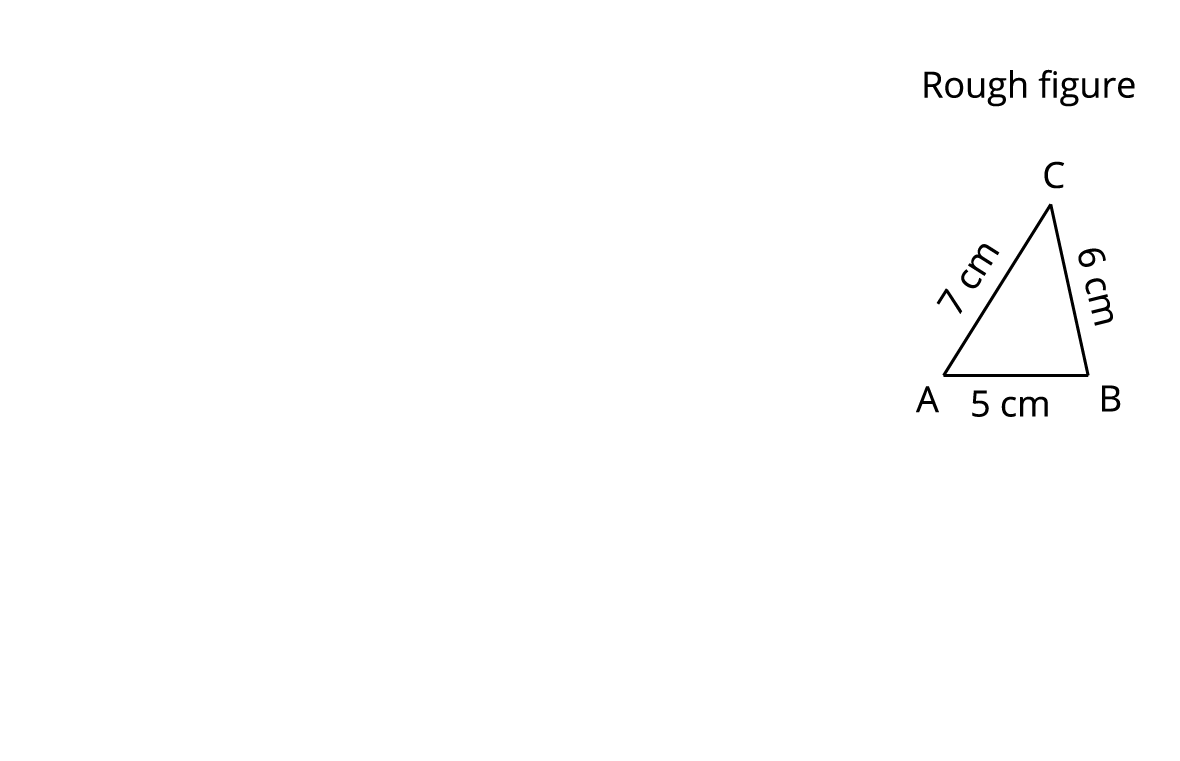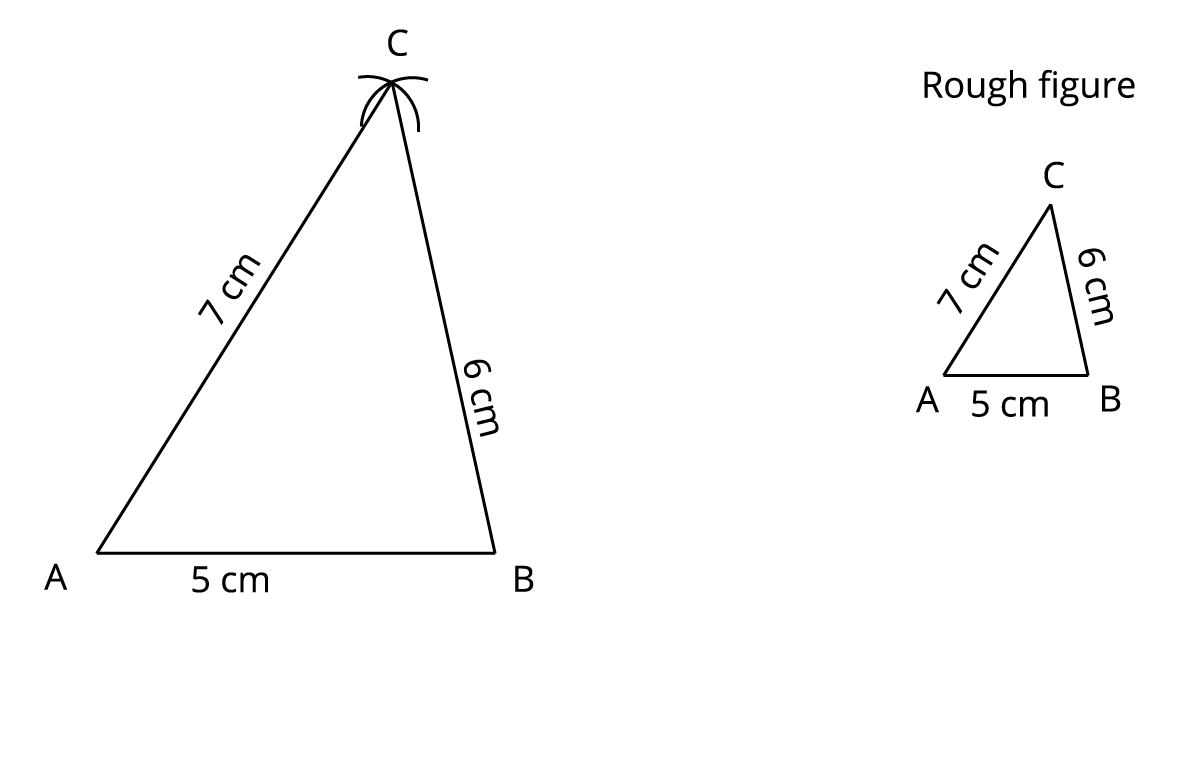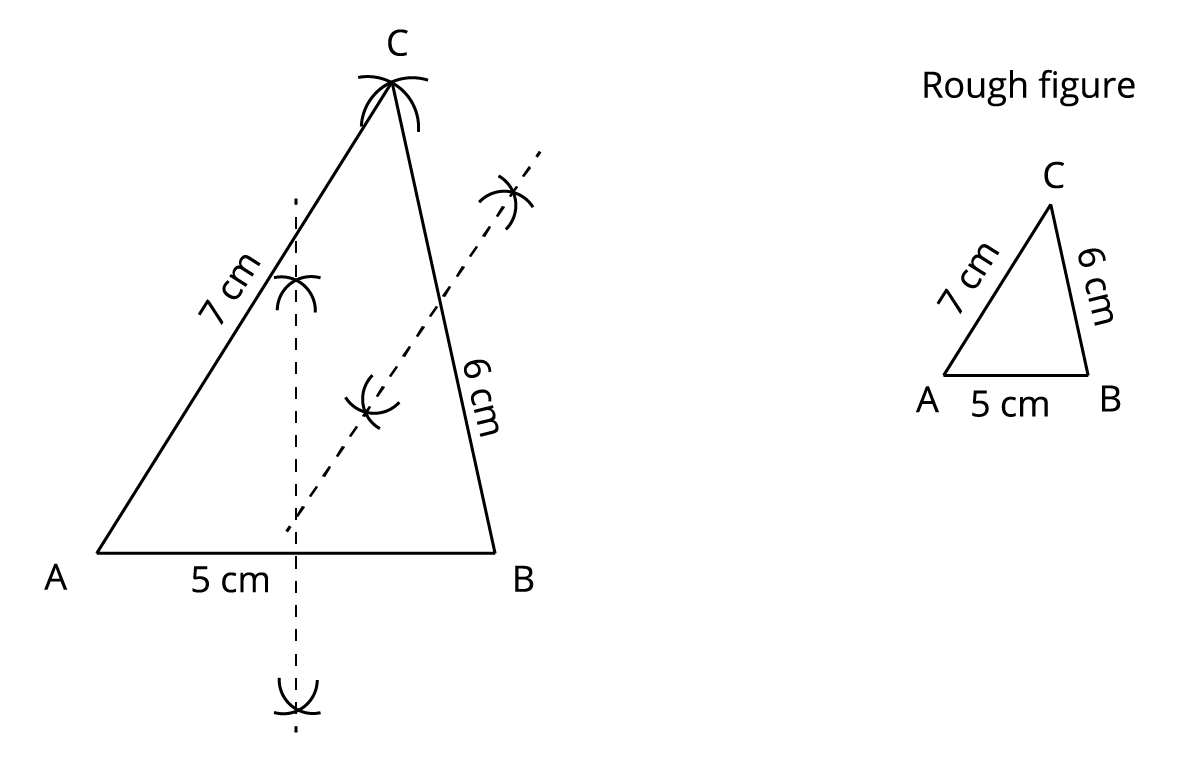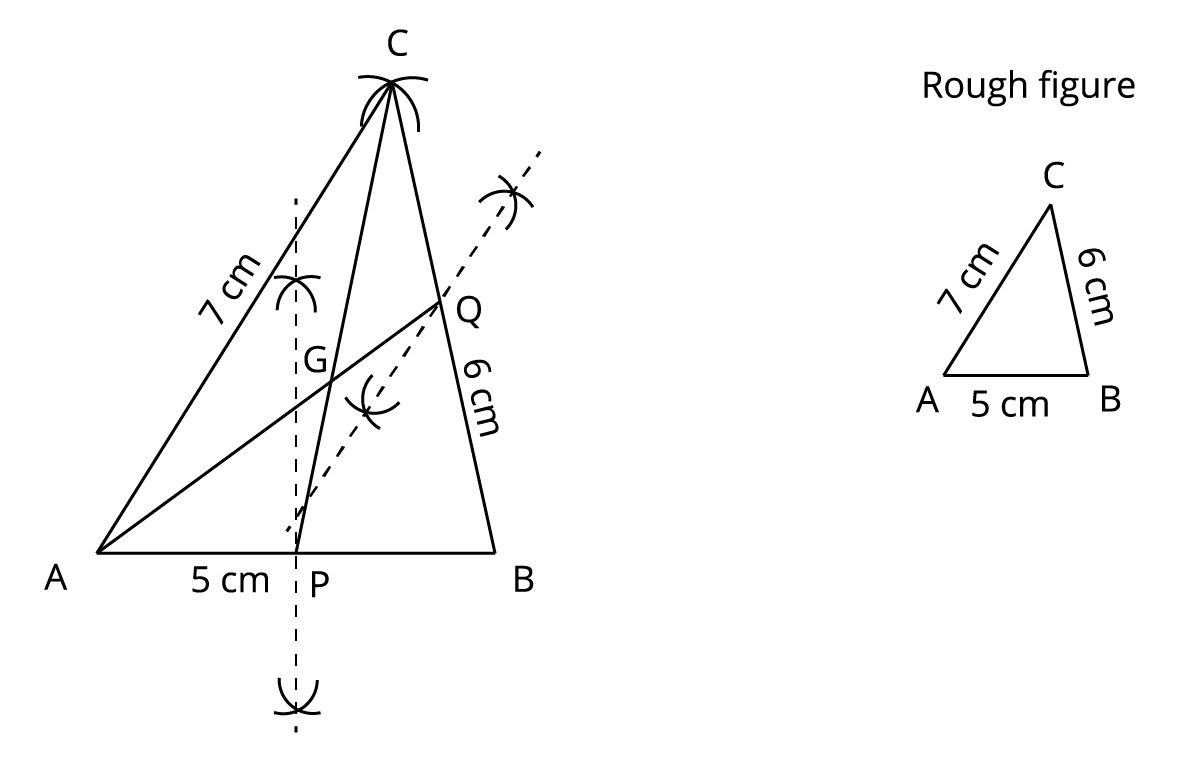UPSKILL MATH PLUS
Learn Mathematics through our AI based learning portal with the support of our Academic Experts!
Learn moreCentroid: It is the point of concurrency of a triangle formed by the intersection of its medians. It is denoted by G.
Working rule to construct the Centroid of a Triangle
Case 1:
Given the length of two sides of a triangle and the measure of one of its interior angle.
Example:
Construct a triangle ABC given AB = 8 cm, BC = 6 cm and \angle BAC = 55^{\circ} and locate its centroid.
Construction:
Step 1: Draw a rough figure for the given question to get the picture of the triangle that is to be constructed.
Step 2: Draw a line segment AB = 8 cm using the ruler.
Step 3: With A as centre, mark an angle 55^{\circ} using the protractor and name it as X. Now join AX.
Step 4: With B as centre, measure 6 cm in the compass and cut an arc intersecting AX and mark it as C.
Step 5: Draw the perpendicular bisectors of any two sides of the triangle AB and BC (say) to find the mid-points of the sides, respectively.
Step 6: Mark the intersecting point of the perpendicular bisectors and the sides AB and BC as P and Q respectively.
Step 7: Draw the medians AQ and CP to meet each other at G, which is the centroid of the triangle.
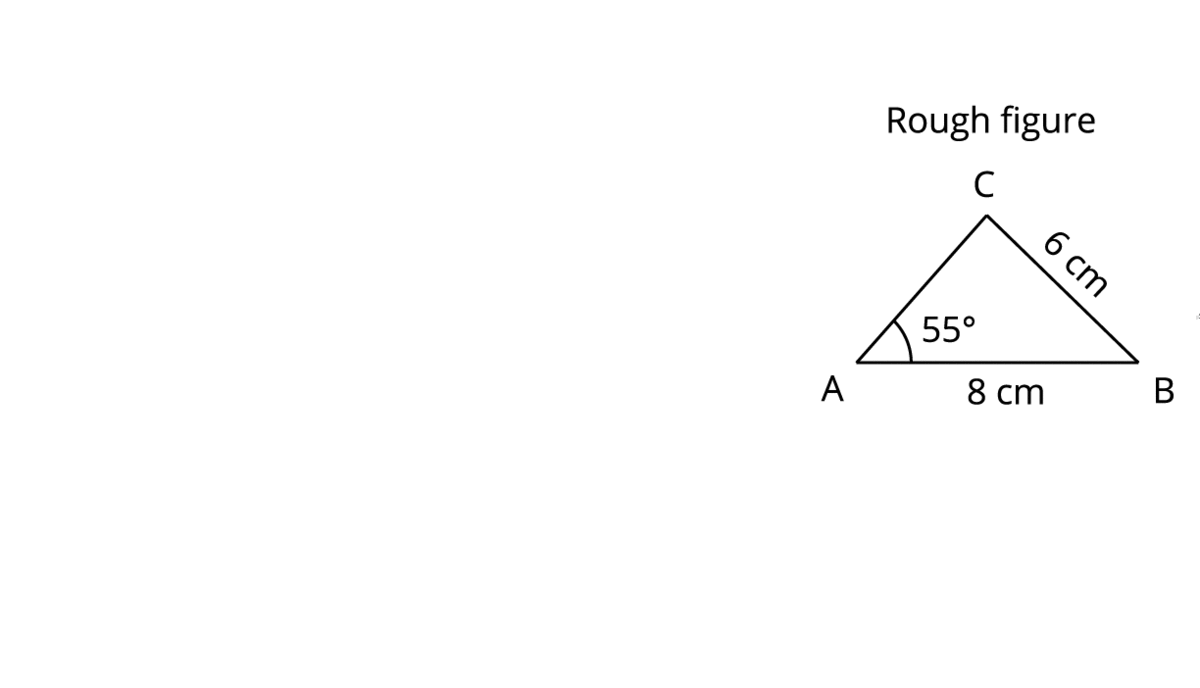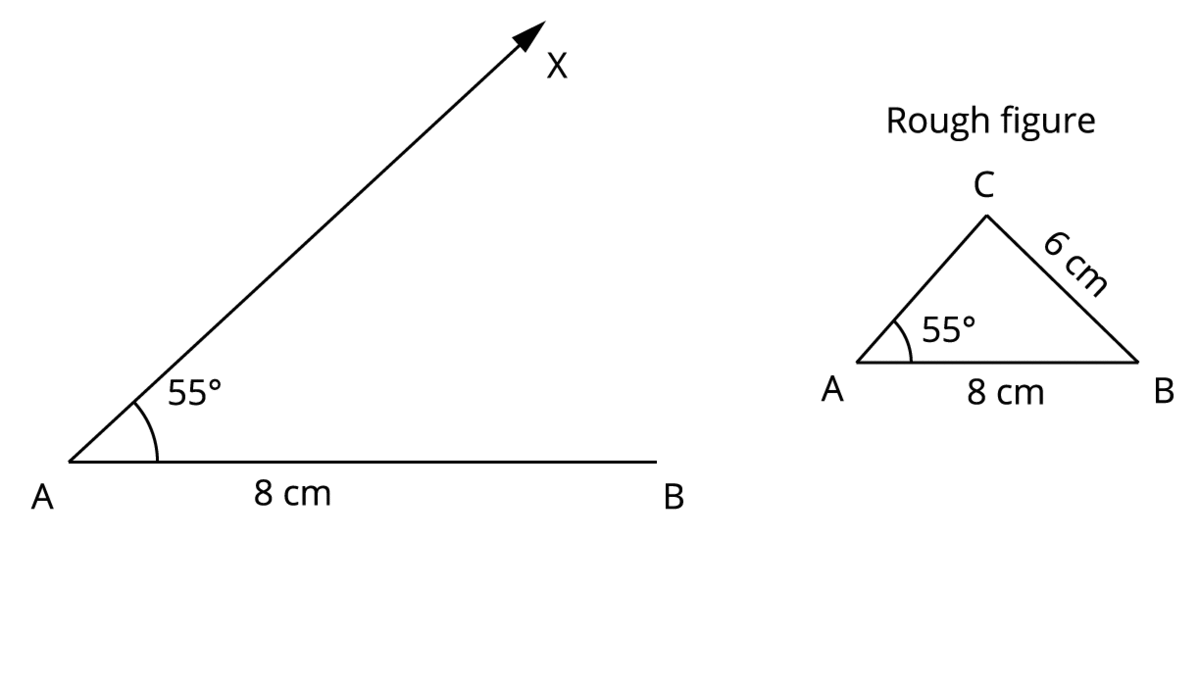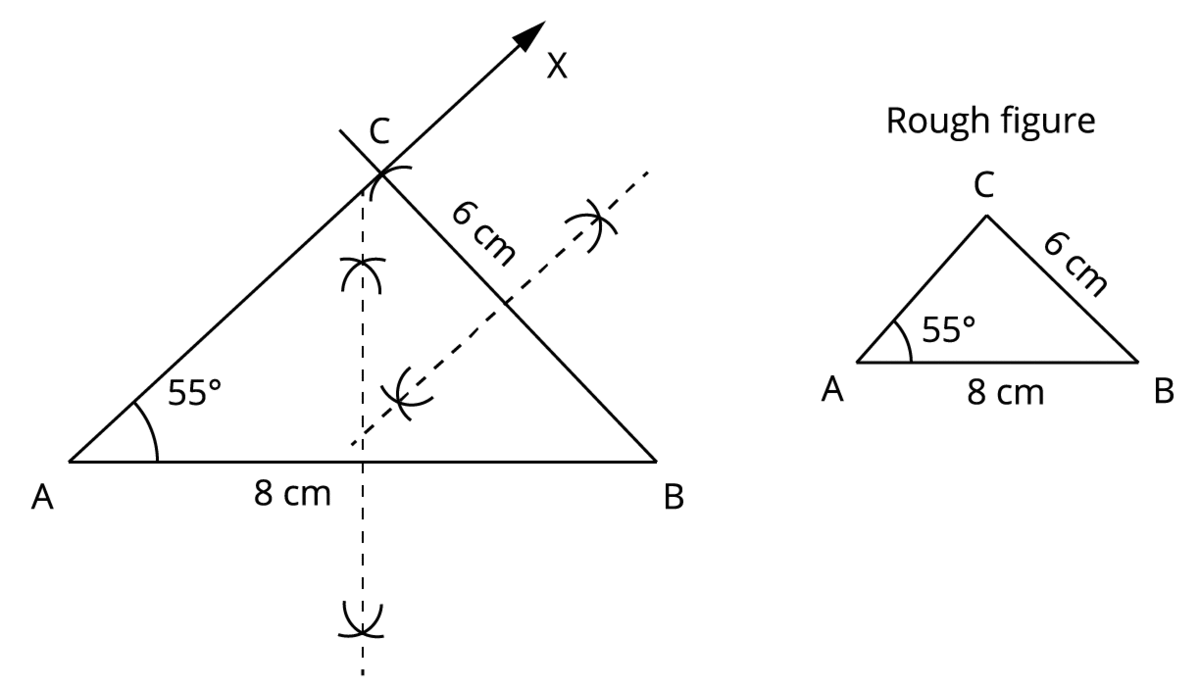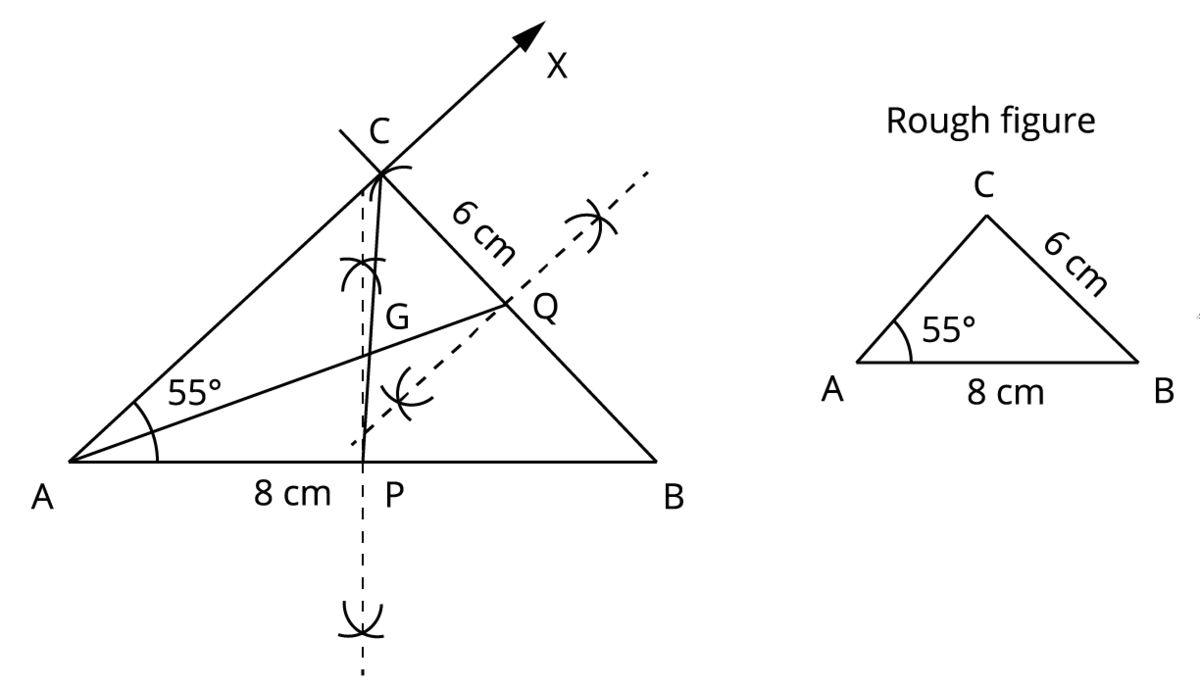
Case 2:
Given the length of all the three sides of a triangle.
Example:
Construct a triangle ABC given AB = 5 cm, BC = 6 cm and CA = 7 cm and locate its centroid.
Construction:
Step 1: Draw a rough figure for the given question to get the picture of the triangle that is to be constructed.
Step 2: Draw a line segment AB = 5 cm using the ruler.
Step 3: With B and A as centre, cut arcs of radius 6 cm and 7 cm respectively to meet each other at C.
Step 4: Draw the perpendicular bisectors of any two sides of the triangle AB and BC (say) to find the mid-points of the sides, respectively.
Step 5: Mark the intersecting point of the perpendicular bisectors and the sides AB and BC as P and Q, respectively.
Step 6: Draw the medians AQ and CP to meet each other at G, which is the centroid of the triangle.