UPSKILL MATH PLUS
Learn Mathematics through our AI based learning portal with the support of our Academic Experts!
Learn moreThere are various types of quadrilaterals. They are:
- Square
- Rectangle
- Parallelogram
- Trapezium
- Rhombus
- Kite
A square is a quadrilateral with four equal sides and four right angles.
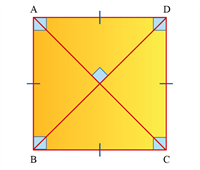
A square has:
- four equal sides \(AB=BC=CD=DA\).
- four right angles \(∠A=∠B=∠C=∠D=90°\).
- two pairs of parallel sides \(AB∥DC\) and \(AD∥BC\).
- two equal diagonals \(AC=BD\).
- diagonals that are perpendicular to each other \(AC⊥BD\).
- diagonals that bisect each other. That is one diagonal divides the other diagonal into exactly two halves.
A rectangle is a quadrilateral with two pairs of equal and parallel opposite sides and four right angles.
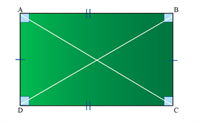
A rectangle has:
- two pairs of parallel sides \(AB∥DC\) and \(AD∥BC\).
- four right angles \(∠A=∠B=∠C=∠D=90°\).
- opposite sides of equal lengths \(AB=DC\) and \(AD=BC\)
- two equal diagonals \(AC=BD\)
- diagonals that bisect each other. That is one diagonal divides the other diagonal into exactly two halves.
A parallelogram is a quadrilateral in which both pairs of opposite sides are parallel.
A parallelogram has:
- two pairs of parallel sides \(PQ∥RT\) and \(PR∥QT\).
- opposite sides of equal lengths \(PQ=RT\) and \(PR=QT\).
- opposite angles that are equal \(∠P=∠T\) and \(∠Q=∠R\).
- two diagonals that bisect each other. That is one diagonal divides the other diagonal into exactly two halves.
A trapezium is a quadrilateral in which one pair of opposite sides is parallel.
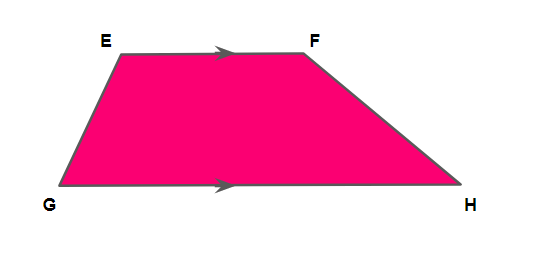
- The sides that are parallel to each other are called bases.
In the above figure, \(EF\) and \(GH\) are bases.
- The sides that are not parallel to each other are called legs.
In the above figure, \(EG\) and \(FH\) are legs.
There is nothing special about the sides, angles, or diagonals of a trapezium.
But if the two non-parallel opposite sides are of equal length, then it is called an isosceles trapezium.
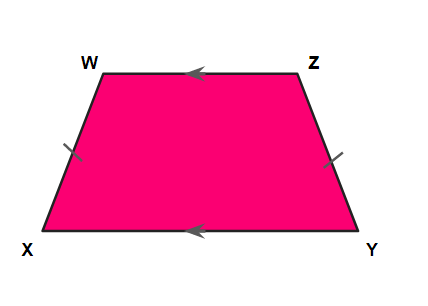
The above quadrilateral \(XYZW\) is an isosceles trapezium.
In an isosceles trapezium, the lengths of the diagonals are equal. That is \(XZ=WY\).
A rhombus is a quadrilateral with four equal sides.
A rhombus has:
- two pairs of parallel sides \(EH∥FG\) and \(EF∥HG\).
- four equal sides \(EH=HG=GF=FE\).
- opposite angles are equal \(∠E=∠G\) and \(∠H=∠F\).
- diagonals that are perpendicular to each other \(EG⊥HF\).
- diagonals that bisect each other. That is one diagonal divides the other diagonal into exactly two halves.
A kite is a quadrilateral in which two pairs of adjacent sides are equal.
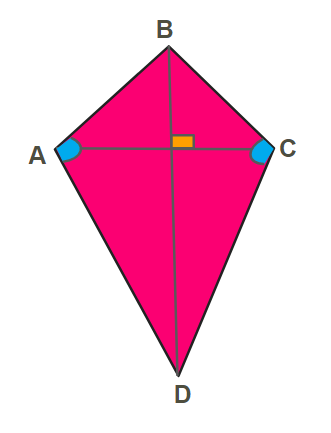
A kite has:
- two pairs of equal adjacent sides \(AB=BC\) and \(CD=DA\).
- one pair of opposite angles (which are obtuse) that are equal \(∠A=∠C\)
- diagonals that are perpendicular to each other \(AC⊥BD\)
- a longer diagonal that bisects the shorter diagonal.
The following chart allows us to understand the hierarchy of quadrilaterals.
Register for free to see more content