
PUMPA - SMART LEARNING
எங்கள் ஆசிரியர்களுடன் 1-ஆன்-1 ஆலோசனை நேரத்தைப் பெறுங்கள். டாப்பர் ஆவதற்கு நாங்கள் பயிற்சி அளிப்போம்
Book Free DemoTheorem III: In parallelogram, opposite angles are equal.
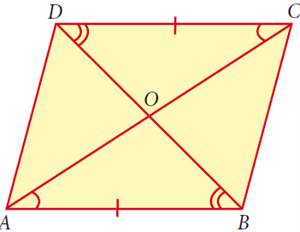
Given: A parallelogram ABCD with AC and BD as its diagonals.
To prove: ∠A = ∠C & ∠B = ∠D.
Proof: We know that 'Opposite sides of a parallelogram is parallel and equal'. So, AB||DC and AD||BC.
Since AB||DC with its transversal line AC.
∠ACD = ∠CAB.
Since AD||BC with its transversal line AC.
∠DAC = ∠BCA.
Thus, \(∠A = ∠DAC+∠CAB = ∠BCA+∠ACD = ∠C\).
Since AB||DC with its transversal line BD.
∠CDB = ∠DBA.
Since AD||BC with its transversal line BD.
∠ADB = ∠DBC.
Thus, ∠B = DBA+∠DBC = ∠CDB+∠ADB = ∠D.
Hence, it is proved.
Theorem IV: The diagonal of a parallelogram bisect each other.

Given: A parallelogram ABCD with AC and BD and O is the point of intesection of AC and BD.
To prove: OA = OC and OB = OD.
Proof: Since 'Opposite sides of a parallelogram is parallel'. So, AB||DC AD||BC.
Since AB||DC & AC is the transversal.
∠ACD = ∠OCD = ∠CAB = ∠OAB (Alternate Interior angle ...1)
That is ∠OCD = ∠OAB.
Since AB||DC & BD is the transversal.
∠BDC = ∠ODC = ∠DBA = ∠OBA (Alternate Interior angle ...1)
That is ∠ODC = ∠OBA.
In \Delta AOB & \Delta COD, two corresponding angles are equal (∠OCD = ∠OAB and ∠ODC = ∠OBA) and a side is equal in measure.
So, OA = OC and OB = OD (Corresponding parts of Congruent triangles)
Hence, it is proved.
Theorem V: Parallelograms on the same base and between the same parallels are equal in area.

Given: Two parallelogram ABCD & ABEF that has same base AB & lies between same parallel CF & AB.
To prove: Area of parallelogram ABCD = Area of parallelogram ABEF
Proof: It can be seen that the parallelogram ABCD and ABEF are on the common base AB.
Here we have a pair of triangle ADF and BCE.
\angle ADF = \angle BCD and \angle AFD \angle BEC.
We know that AD = BC and AF =BE (Opposite sides are equal in measure).
Thus, two of the sides and two of the angles are equal.
By AAS congruence, the triangles ADF and BCE are congruent triangles.
We know that the congruent triangles have the same area.
Now, area of parllelogram ABCD = Area of \Delta BCE + Area of quadrilateral ABED.
= \Delta BCE+ Area of parallelogram ABED
= Area of parallelogram ABEF
Area of parllelogram ABCD = Area of parallelogram EFCD
Hence, it is proved.
In this process, we have also proved other interesting statements. These are called Corollaries, which do not need separate detailed proofs.
Important!
Corollary 1: Triangles on the same base and between the same parallels are equal in area.
Corollary 2: A rectangle and a parallelogram on the same base and between the same parallels are equal in area.