PUMPA - SMART LEARNING
எங்கள் ஆசிரியர்களுடன் 1-ஆன்-1 ஆலோசனை நேரத்தைப் பெறுங்கள். டாப்பர் ஆவதற்கு நாங்கள் பயிற்சி அளிப்போம்
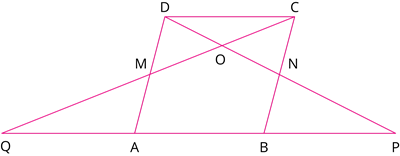
Book Free DemoIn parallelogram \(ABCD\) of the accompanying diagram, line \(DP\) is drawn bisecting \(BC\) at \(N\) and meeting \(AB\) (extended) at \(P\). From vertex \(C\), line \(CQ\) is drawn bisecting side \(AD\) at \(M\) and meeting \(AB\) (extended) at \(Q\). Lines \(DP\) and \(CQ\) meet at \(O\). Show that the area of triangle \(QPO\) is \(\frac{9}{8}\) of the area of the parallelogram \(ABCD\).
In \(\Delta QAM\) and \(\Delta CDM\):
\(\angle QMA = \angle\) [vertical angles]
[Since \(M\) is a mid point]
\(\angle CDM = \angle \) [Since \(CD || QA\) and \(AD\) is a transversal]
Thus, \(\Delta \) \(\cong \Delta CDM\) [by congruence criterion] - - - (1)
Similarly, \(\Delta PBN \cong \Delta \) [by congruence criterion] - - - (2)
Now, Area of \(\Delta QPO\) \(=\) Area of \(\Delta QAM\) \(+\) Area of \(+\) Area of \(\Delta PBN\)
\(=\) Area of \(\Delta CDM\) \(+\) Area of \(+\) Area of \(\Delta DCN\)
\(=\) Area of \(+\) Area of \(\Delta CDM\) \(+\) Area of \(\Delta CON\) \(+\) Area of \(\Delta COD\)
\(=\) Area of \(ABCD\) \(+\) Area of \(\Delta \) - - - (3)
Area of \(\Delta COD\) \(=\) \(\frac{1}{4} \times\) Area of
\(=\) \(\frac{1}{4} \times \frac{1}{2}\) Area of
\(=\) \(\frac{1}{8}\) Area of - - (4)
Substitute equation (4) in equation (3).
Area of \(\Delta QPO\) \(=\) Area of \(ABCD\) \(+\) \(\frac{1}{8}\) Area of
\(=\) \(1 + \frac{1}{8}\) (Area of \(ABCD\) \(+\) Area of )
\(=\)\(\frac{9}{8}\) Area of
Hence proved.
Register for free to see more content