PDF chapter test TRY NOW
The decimal expansion of an irrational number is non-terminating and non-recurring. Conversely, the decimal expansion of a number is non-terminating and non-recurring is an irrational number.
Example:
Many square roots and cube roots are irrational numbers.
Let us find the square root of .
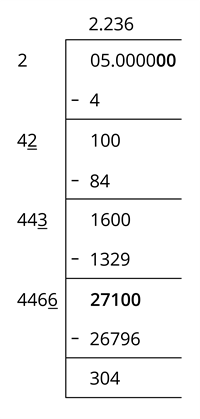
Thus, the decimal expansion of have non-terminating and non-recurring decimals.
It goes like
Some of the famous irrational numbers:
Irrational Number | Its non-terminating and non-recurring decimal value |
| \(3.141592653589793\)... | |
| \(2.718281828459045\)... | |
| \(1.618033988749894\)... |
Register for free to see more content