UPSKILL MATH PLUS
Learn Mathematics through our AI based learning portal with the support of our Academic Experts!
Learn moreDifference of two sets:
The difference of sets \(A\) and \(B\) is the set of elements which belong to \(A\) but not to \(B\). Symbolically, we write \(A – B\) and read as "\(A\) minus \(B\)".
Symbolically we write \(A-B=\{x:x\in A\ \mathrm{and}\ x\notin B\}\) and \(B-A=\{x:x\notin A\ \mathrm{and}\ x\in B\}\)
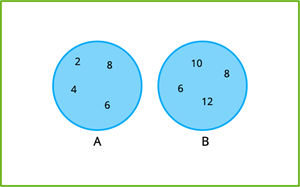
Let \(A=\{2,\ 4,\ 6,\ 8\}\), \(B=\{6,\ 8,\ 10,\ 12\}\). Find \(A-B\) and \(B-A\).
In the Venn diagram below \(A\) and \(B\) are represented.
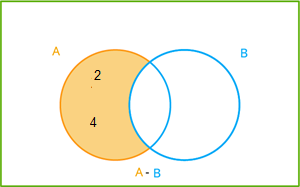
In the Venn diagram below, the area in the yellow colour represents \(A - B\).
In the Venn diagram below, the area in the yellow colour represents \(B - A\).
Symmetric Difference of Sets:
The symmetric difference of two sets \(A\) and \(B\) is the set of union elements which belong to \(A\) but not to \(B\), and symbolically we write \(A – B\) and read as "\(A\) minus \(B\)". If it belong to \(B\) but not to \(A\), symbolically we write \(B – A\) and read as "\(B\) minus \(A\)". Symbolically we write \((A–B)∪(B–A)\). It is denoted by \(A Δ B\).
Symbolically we write \(A\Delta B = \{x:x\in A-B\ \mathrm{or}\ x\in B-A\}\).
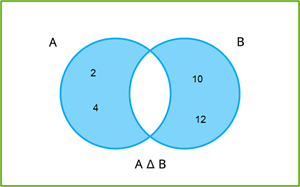
In the Venn diagram below \(A\) and \(B\) are represented.
In the Venn diagram below, the area in the blue colour represents \(A Δ B\).
Complement of a set:
Let \(U\) be the universal set, and \(A\) be a subset of \(U\). Then the complement of \(A\) is the set of all elements of \(U\) which are not the elements of \(A\). Symbolically, we write \(A′\) to denote the complement of \(A\) with respect to \(U\).
Symbolically we write \(A^\prime = \{x: x\in U\ \mathrm{and}\ x\notin A\}\). Obviously \(A^\prime=U-A\).
Note: The complement of a set \(A\) can be looked upon, alternatively, as the difference between a universal set \(U\) and the set \(A\).
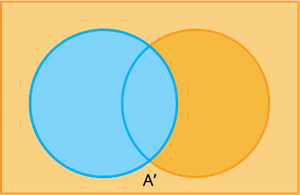
In the Venn diagram below, the area in the blue colour represents \(A'\).
If \(A\) and \(B\) are two sets, then the complement of \(A\) and \(B\) are \(A' = U - A\) and \(B' = U - B\)
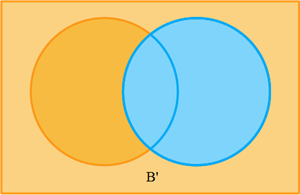
In the Venn diagram below, the area in the blue colour represents \(A'\).
In the Venn diagram below, the area in the blue colour represents \(B'\).
Register for free to see more content