PDF chapter test TRY NOW
We know that a ray of light travelling obliquely from one transparent medium to another will change direction in the second medium.
The term refractive index can be used to express the magnitude of the change in direction that occurs in a given pair of media. The relative speed of light propagation in different media can be linked to the refractive index, an important physical quantity. Light propagates at different speeds in different media, as it turns out.
In a vacuum, light travels at the fastest speed. When compared to vacuum, the speed of light in air is only slightly slower. In glass or water, it shrinks dramatically.
The value of the refractive index for a given pair of media is determined by the speed of light in the two media.
Consider a light ray passing through medium 1 and medium 2.
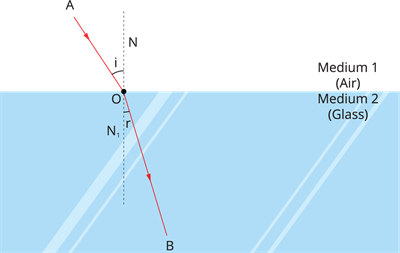
Two medium with interface
Let be the light speed in medium 1 and be the light speed in medium 2.
The ratio of the speed of light in medium 1 to the speed of light in medium 2 gives the refractive index of medium 2 compared to medium 1. The symbol is commonly used to represent it.
This can be expressed mathematically as,
By the same argument, the refractive index of medium 1 with respect to medium 2 is represented as . It is given by
When medium 1 is vacuum or air, the refractive index of medium 2 is taken into account in relation to vacuum. This is referred to as the medium's absolute refractive index. is the simplest way to express it. If c is the speed of light in air and v is the speed of light in the medium, then the medium's refractive index is n_m.
A medium's absolute refractive index is simply referred to as its refractive index.
The refractive index of water is = 1.33.
This means that the ratio of light speed in air to light speed in water is equal to 1.33.
Similarly, crown glass has a refractive index of = 1.52.
Points to know:
The optical density of a medium is also a measure of its ability to refract light. Optical density has a specific meaning. It is not to be confused with mass density. In this chapter, we have used the terms rarer medium and denser medium. It actually stands for optically rarer medium and optically denser medium. When is it appropriate to say that one medium is optically denser than another? When two media are compared, the one with the higher refractive index is optically denser than the other.
The optically rarer medium has a lower refractive index. A rarer medium has a faster speed of light than a denser medium. As a result, a ray of light moving from a rarer to a denser medium slows down and bends towards the normal. It speeds up and bends away from the normal as it moves from a denser medium to a rarer medium.
