13. Linear expansion

Linear expansion in solid

The increase in the length is proportional to the rise in temperature, original length, and material type.

The amount by which the unit length of a material increases when the temperature is raised one degree is called the coefficient of linear expansion. The unit of coefficient of linear expansion is ${}^{o}k^{-}{}^1$. The value of the coefficient of linear expansion is different for different materials.

The coefficient of linear expansion is used to find out the actual increase in the length. It can be represented by the symbol $\mathrm{\alpha }$ (alpha).

Consider a material with the length of $l_1$ at $t_1^o$ \(c\). The material is heated up to $t_2^o$ \(c\). Now, the length of the material is $l_2$.

Let $\mathrm{\alpha }$ be the coefficient of linear expansion.

We know that,

The change in length \(ΔL\) is proportional to the original length $l_1$, rise in temperature \(ΔT\), and material type.

$\begin{array}{l}\mathrm{\Delta }L=l_2-l_1\mathit{and}\\ \\ \mathrm{\Delta }T=t_2-t_1\\ \\ \mathrm{\Delta }L\propto l_1\mathrm{\Delta }T\\ \\ \mathrm{\Delta }L=\mathrm{\alpha }{l}_1\mathrm{\Delta }T\end{array}$

Rearranging the above equation,

 $\mathrm{\alpha }=\frac{l_2-l_1}{l_1\left(t_2^o-t_1^o\right)}$ ----  eqn 1

The $\mathrm{\alpha }$ will vary for different materials.

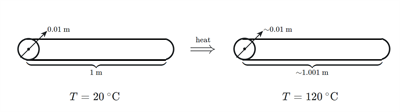
In above diagram,

 $l_1$ = \(1\) \(m\)

 $t_1^o$ = ${20}^o$\(c\)

$t_2^o$ = ${120}^o$\(c\)

$l_2$ = \(1.001\) \(m\)

then, $\mathrm{\alpha }=\frac{l_2-l_1}{l_1\left(t_2^o-t_1^o\right)}$ 

$\mathrm{\alpha }=\frac{1.001-1}{1.001\left(120-20\right)}$ = \(0.0000099\)${}^{o}k^{-}{}^1$.

We shall calculate the length after the expansion using the equation.

Change (increase) in length,

$\begin{array}{l}{l}_2=l_1(1+\mathrm{\alpha }t)\\ l_c=l_1\times \mathrm{\alpha }\times t\\ \mathit{where},\\ l_2=\mathit{length}\mathit{after}\mathit{expansion}\\ l_c=\mathit{Change}\mathit{in}\mathit{length}\\ t=\left(t_2-t_1\right)\end{array}$: