PDF chapter test TRY NOW
Volume is a space occupied by any three-dimensional object. It is also a derived quantity that can be measured by measuring lengths.
The formula is written as,
SI Unit
If the unit of volume is written in m, then
Volume = metre × metre × metre
= cubic\ metre or m^3
Hence, the SI unit of volume is a cubic metre (or) m^3.
The volume of regularly shaped objects
The volume of regularly shaped figures can be calculated using the relevant formula. Some of them are tabulated below.
Shape | Three-dimensional figure | Volume |
Cube |  | |
Cuboid | 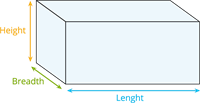 | |
Sphere |  | |
Cylinder | 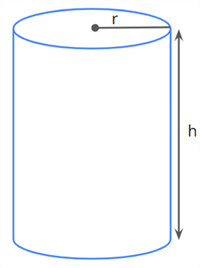 |
Solved examples
1. Calculate the volume of a cube having sides of 10\ m.
Sides, a\ = 10\ m
Sides, a\ = 10\ m
By substituting the known values on the formula of a cube, we get the following.
Therefore, the volume of a cube is 1000\ m^3.
2. What will be the volume of a cylinder having a 2\ m radius and 7\ cm height?
Radius, r\ = 2\ m
Height, h\ = 7\ m
Therefore, the volume of a cylinder is 1000\ cm^3.
Reference:
https://upload.wikimedia.org/wikipedia/commons/d/df/Simple_sphere_with_radii.svg
