PDF chapter test TRY NOW
There are three spheres \(A\), \(B\), \(C\) as shown below:
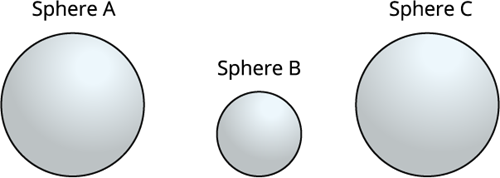
Three spheres
Sphere \(A\) and \(B\) are made of the same material. Sphere \(C\) is made of a different material. Spheres \(A\) and \(C\) have equal radii. The radius of sphere \(B\) is half that of \(A\). Density of \(A\) is double that of \(C\).
Now answer the following questions:
i. Find the ratio of masses of spheres \(A\) and \(B\).
Let the mass of spheres \(A\) and \(B\) be \(M_A\) and \(M_B\), respectively.
Let the volume of spheres \(A\) and \(B\) be \(V_A\) and \(V_B\), respectively.
Let the volume of spheres \(A\) and \(B\) be \(V_A\) and \(V_B\), respectively.
Let the ratio of masses of spheres \(A\) and \(B\) be \(M_A\) : \(M_B\).
Density is denoted as \(D\).
The ratio of sphere is given as .
ii. Find the ratio of volumes of spheres \(A\) and \(B\).
Since mass is to volume, the ratio of volumes of spheres \(A\) and \(B\) is .
iii. Find the ratio of masses of spheres \(A\) and \(C\).
The ratio of masses of spheres \(A\) and \(C\) is given as . Therefore, the density of \(A\) is double that of \(C\).
Register for free to see more content