
PUMPA - SMART LEARNING
எங்கள் ஆசிரியர்களுடன் 1-ஆன்-1 ஆலோசனை நேரத்தைப் பெறுங்கள். டாப்பர் ஆவதற்கு நாங்கள் பயிற்சி அளிப்போம்
Book Free DemoThe Aeroplane, aircraft and ships not only have to know their speed but also their direction. Take a ship as an example; it has to maintain its speed and direction. And that's where the concept of average velocity comes into play. Similar to the average speed, we can also define average velocity.
Average velocity:
Average velocity can be defined as the ratio between the total displacement and the total time taken.
Now, we will learn how to find the average velocity of a vehicle.
Consider a taxi driver travels 9 km in 6 minutes to drop a passenger, then he reverses his directions and drives 3km in 5 minutes to pick up another passenger. Now, calculate the average velocity of the taxi.
We know that velocity \(=\) Total displacement / Total time taken
Total displacement \(=\) 9 \(km -\) 3 \(km =\) 6 km.
Total time taken \(=\) 6 minutes \(+\) 5 minutes \(=\) 11 minutes.
Therefore, average velocity \(=\) km/minutes.
Zero Displacement:
In some circumstances, the displacement can be zero. Let's see an example to understand this clearly.
Consider that you're a formula one race car driver; you finished a 250 km race in one hour and thirty minutes. You finished at the place where you started.
Then what will be your average velocity?
We know that average velocity is .
First, we have to find the displacement. But, the important thing is your starting and finishing point are the same. Therefore, the displacement will be zero.
If the displacement is zero, then the average velocity is also zero.
Important!
Note: If the initial and final point of an object is in the same quantity, then the displacement is zero. Because there is no displace occurred.
Relation between \(v\), \(d\) and \(t\).
The below triangle diagram can help you to recall the relationship between velocity (\(v\)), displacement (\(d\)), and time(\(t\)).
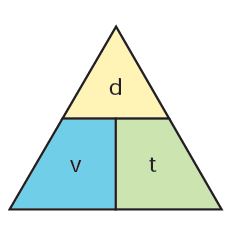
The relation is,
Therefore, simply we can write, .