PDF chapter test TRY NOW
Statement:
The lengths of tangents drawn from an exterior point to a circle are equal.
Proof for the theorem:
Consider a circle with centre \(O\).
Let \(PA\) and \(PB\) be the two tangents drawn from the external point \(P\) to the circle.
Construction:
Join \(OA\), \(OB\) and \(OP\).
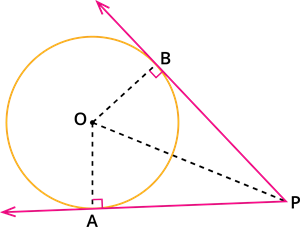
To prove:
The tangent \(PA\) \(=\) The tangent \(PB\)
Proof:
By the theorem \(1\), we have:
The tangent at any point of a circle is perpendicular to the radius through the point of contact.
\(OB\) \(\perp\) \(PB\) and \(OA\) \(\perp\) \(PA\).
Here, \(OA\) and \(OB\) are radius. Hence, they are equal.
The side \(OP\) is a common side to the triangles \(AOP\) and \(BOP\).
Therefore, by the RHS rule (In two right-angled triangles, if the length of the hypotenuse and one side of one triangle is equal to the length of the hypotenuse and corresponding side of the other triangle, then the two triangles are congruent.), the triangles \(AOP\) and \(BOP\) are congruent.
We know that the corresponding parts of the congruent triangles are equal.
Therefore, \(PA = PB\).
Example:
In the above given figure if \(OB\) \(=\) \(3\) \(cm\) and \(OP\) \(=\) \(5\) \(cm\), find the length of \(PA\).
Solution:
By the theorem \(1\), we have:
A tangent at any point on a circle and the radius through the point are perpendicular to each other.
\(\angle OPB\) \(=\) \(90^{\circ}\).
So, \(OPB\) is a right angled triangle.
By the Pythagoras theorem, we have:
In a right triangle, the square of the hypotenuse is equal to the sum of the squares of the other two sides.
\(OP^2\) \(=\) \(OB^2\) \(+\) \(PB^2\)
\(PB^2\) \(=\) \(OP^2\) \(-\)\(OB^2\)
\(PB^2\) \(=\) \(5^2\) \(-\) \(3^2\)
\(PB^2\) \(=\) \(25 - 9\)
\(PB^2\) \(=\) \(16\)
\(\Rightarrow\) \(PB\) \(=\) \(\sqrt{16}\)
\(PB\) \(=\) \(4\)
Thus, the measure of \(PB\) \(=\) \(4\) \(cm\)
By the theorem \(2\), we have:
The lengths of the two tangents drawn from an exterior point to a circle are equal.
Hence, \(PA\) \(=\) \(PB\).
Therefore, the measure of \(PA\) \(=\) \(4\) \(cm\)
Important!
In the above given figure, \(\angle OPA\) \(=\) \(\angle OPB\) where \(OP\) is the angle bisector of \(\angle APB\).
Register for free to see more content