A monomial and its standard form — lesson. Mathematics CBSE, Class 7.

PUMPA - SMART LEARNING

எங்கள் ஆசிரியர்களுடன் 1-ஆன்-1 ஆலோசனை நேரத்தைப் பெறுங்கள். டாப்பர் ஆவதற்கு நாங்கள் பயிற்சி அளிப்போம்

Book Free Demo

A product of numbers, variables and their exponents is called a monomial.

Example:

x \cdot x = x^{2}

a \cdot a \cdot b = a^{2} b

\begin{array}{l} 3 x \cdot 5 x = (3 \cdot 5) \cdot (x \cdot x) = \\ = 15 x^{2} \end{array}

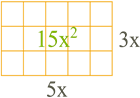
Important!

Typically, we won't keep multiple signs between numerical factors and variables (

6 \cdot a \cdot y

$= 6ay$)

Important!

Note that one variable, such as $x$, is also considered a monomial, because

x = 1 \cdot x

. A number like $3$ is also a monomial, because

3 = 3 \cdot x^{0}

Some monomials can be simplified.

Example:

Let's simplify the monomial

(6 x y^{2} \cdot (- 2) x^{3} y)

using the exponents' multiplication rule:

a^{m} \cdot a^{n} = a^{m + n}

(6 x y^{2} \cdot (- 2) x^{3} y)

$=$

6 \cdot (- 2) x x^{3} y^{2} y = - 12 x^{4} y^{3}

(numerical factors are multiplied, but the exponents of the variables are added)

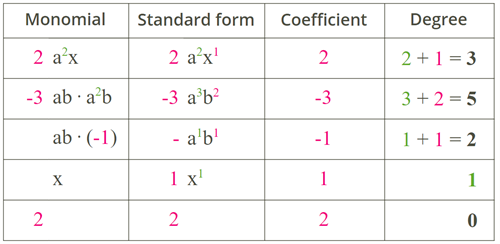
Standard form of a monomial

A monomial is in a standard form, if first there is a constant (numerical) factor, and then variable factors in alphabetical order. If there are several variable factors with the same base, you multiply them, thus you get one factor for each variable.

A monomial is in a standard form, if:

every product of the same variables is written as one variable with an exponent $a^{m} \cdot a^{n} = a^{m + n}$ ;
a constant factor (or the monomial coefficient) is written as the first term of a monomial.