PUMPA - SMART LEARNING
எங்கள் ஆசிரியர்களுடன் 1-ஆன்-1 ஆலோசனை நேரத்தைப் பெறுங்கள். டாப்பர் ஆவதற்கு நாங்கள் பயிற்சி அளிப்போம்
Book Free DemoLet's learn the types of triangle:
Triangles can be classified based on its sides as well as its angles.
Types of triangles based on its side lengths are as follows.
- Equilateral triangle
- Isosceles triangle
- Scalene triangle
A triangle is an equilateral when all its sides are equal in length.
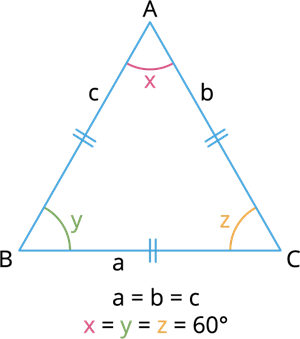
Example:
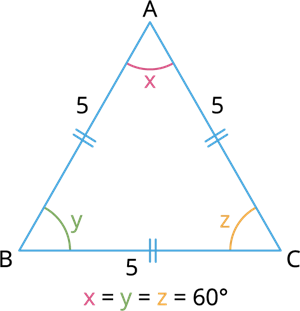
In here, the sides are equal in measure AB=BC=CA=5 units.
A triangle is isosceles when two of its sides are equal in length.
Example:
In here, the two of the sides are equal in measure AB=CA=1 unit and BC=√2 units.
A triangle is a scalene when all the three sides are unequal.
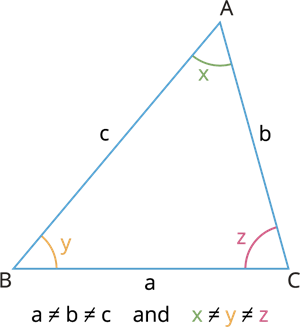
Example:
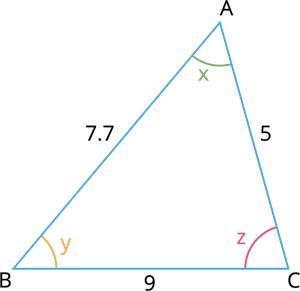
In here, all the sides are unequal in measure AB=7.7, CA=5 units and BC=9 units.
Types of triangles based on its angles are as follows.
- Acute angled triangle
- Right angle triangle
- Obtuse angled triangle
A triangle is acute angle triangle when all the three of its angle are acute (less than 90°).
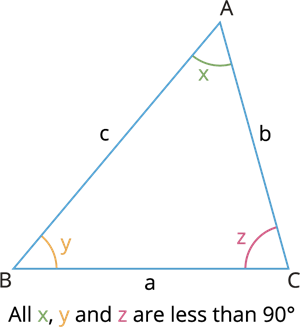
Example:
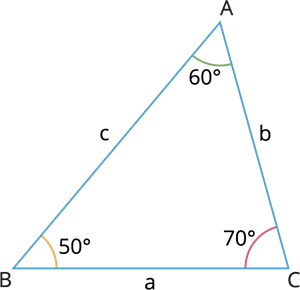
In here, all the three angles are less than 90°. That is, ∠A=60°, ∠B=50° and ∠C=70°.
A triangle is right angle triangle when one of its angles is a right angle (90°), and the other two are acute angles (less than 90°).
Example:
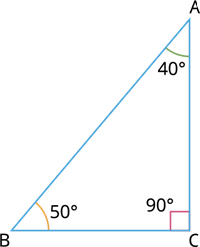
In here, one among the angle is 90°, and the other two are acute. That is, ∠A=40°, ∠B=50° and ∠C=90°.
A triangle is an obtuse angle triangle when one of its angles is an obtuse angle (greater than 90°), and the other two are acute angle (less than 90°).
Example:
In here, one among the angle greater than 90° and the other two are acute. That is, ∠A=120°, ∠B=35° and ∠C=25°.
Register for free to see more content