UPSKILL MATH PLUS
Learn Mathematics through our AI based learning portal with the support of our Academic Experts!
Learn moreConjecture 1
Illustration:
Consider a circle with centre O and a diameter QR.
Place a points P on the circumference of the circle.

It is known that the angle measure of a diameter in a circle is 180^{\circ}.
This implies, the arc subtends an \angle QOR = 180^{\circ} at the centre O and \angle QPR at the circumference of the circle.
According to the theorem, the angle subtended by an arc of the circle at the centre is double the angle subtended by it at any point on the remaining part of the circle.
So, \angle QOR = 2\angle QPR.
This implies, \angle QPR = \frac{1}{2} \times \angle QOR
= \frac{180^{\circ}}{2}
= 90^{\circ}
Based on this result, we arrive at a conjecture which states:
An angle inscribed in a semi-circle is a right angle.
Explanation:

The conjecture is that any angle inscribed in the semi-circle is always 90^{\circ}. In other words, the angle subtended by the diameter is always 90^{\circ}.
Example:
Find the measure of the angle subtended by the longest chord of the circle at any point on the circumference.
Solution:
The longest chord of the circle is the diameter of the circle.
Also, the angle subtended by the diameter of the circle at any point on the circumference is the same as the angle inscribed in a semi-circle.
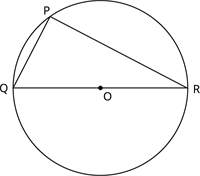
Let QR be the diameter and P be any point on the circumference.
It is known that an angle inscribed in a semi-circle is a right angle.
This implies, \angle QPR = 90^{\circ}.
Therefore, the measure of the angle subtended by the longest chord of the circle at any point on the circumference is 90^{\circ}.
Conjecture 2
Equal arcs of a circle subtend equal angles at the centre.
Explanation:
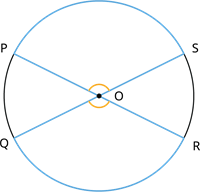
The conjecture is that if the arcs and are equal, then the angles subtended by them at the centre are equal. \angle POS = \angle QOR.
Example:
If and are two equal arcs and if one of the arcs subtend an angle 60^{\circ} at the centre, then find the angle measure of the other arc at the centre.
Solution:
It is known that equal arcs of a circle subtend equal angles at the centre.
Hence, it is obvious that the other arc subtends an angle 60^{\circ} at the centre.