Theorem I, II and III — lesson. Mathematics CBSE, Class 9.

PUMPA - SMART LEARNING

எங்கள் ஆசிரியர்களுடன் 1-ஆன்-1 ஆலோசனை நேரத்தைப் பெறுங்கள். டாப்பர் ஆவதற்கு நாங்கள் பயிற்சி அளிப்போம்

Book Free Demo

Theorem I:

A diagonal of a parallelogram divides it into two congruent triangles.

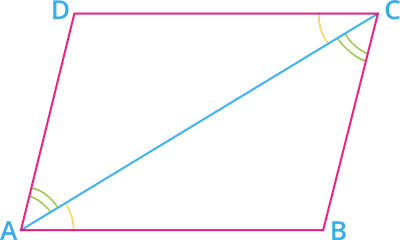
Given:

$ABCD$ is a parallelogram, and

$AC$ is a diagonal.

Diagonal divides the quadrilateral into two triangles

$ABC$ and

$ADC$ .

To prove:

$\Delta ABC \cong \Delta CDA$

Proof: We know that "the opposite sides of a parallelogram are parallel".

$AB \ || \ DC$ and

$BC \ || \ AD$

Since

$AB \ || \ DC$ and

$AC$ is a transversal,

$\angle BAC = \angle DCA$ [alternate interior angles] - - - - - (I)

Also,

$BC \ || \ AD$ and

$AC$ is a transversal,

$\angle BCA = \angle DAC$ [alternate interior angles] - - - - - (II)

$\Delta ABC$ and

$\Delta CDA$ :

$\angle BAC = \angle DCA$ [from (I)]

$\angle BCA = \angle DAC$ [from (II)]

$AC = AC$ [Common side]

Thus,

$\Delta ABC \cong \Delta CDA$ [by

$ASA$ congruence rule].

Therefore, the diagonal of a parallelogram divides it into two congruent triangles.

Theorem II:

In a parallelogram, opposite sides are equal.

Given:

$ABCD$ is a parallelogram, and

$AC$ is a diagonal.

Diagonal divides the quadrilateral into two triangles

$ABC$ and

$ADC$ .

To prove:

$AB = CD$ and

$BC = AD$

Proof: We know that "the opposite sides of a parallelogram are parallel".

$AB \ || \ DC$ and

$BC \ || \ AD$

Since

$AB \ || \ DC$ and

$AC$ is a transversal,

$\angle BAC = \angle DCA$ [alternate interior angles] - - - - - (I)

Also,

$BC \ || \ AD$ and

$AC$ is a transversal,

$\angle BCA = \angle DAC$ [alternate interior angles] - - - - - (II)

$\Delta ABC$ and

$\Delta CDA$ :

$\angle BAC = \angle DCA$ [from (I)]

$\angle BCA = \angle DAC$ [from (II)]

$AC = AC$ [Common side]

Thus,

$\Delta ABC \cong \Delta CDA$ [by

$ASA$ congruence rule].

Corresponding parts of congruent triangles are equal.

$\Rightarrow AB = DC$ and

$BC = AD$ .

Therefore, the opposite sides of a parallelogram are equal.

Theorem III:

If each pair of opposite sides of a quadrilateral is equal, then it is a parallelogram.

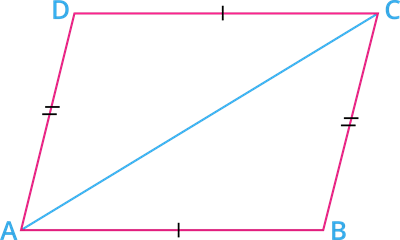
Given:

$ABCD$ is a quadrilateral, where

$AB = DC$ and

$BC = AD$ .

Construction: Join the diagonal

$AC$ .

To prove:

$ABCD$ is a parallelogram.

Proof: In

$\Delta ABC$ and

$\Delta CDA$ :

$AB = DC$ [Given]

$BC = AD$ [Given]

$AC = AC$ [Common side]

Thus,

$\Delta ABC \cong \Delta CDA$ [by

$SSS$ congruence rule].

$\implies \angle BAC = \angle DCA$ [by CPCT] - - - - (I)

$\implies \angle BCA = \angle DAC$ [by CPCT] - - - - (II)

These angles are alternate interior angles.

We know that "the alternate interior angles are equal only when the lines are parallel".

So,

$AB \ || \ DC$ and

$BC \ || \ AD$ .

Hence,

$ABCD$ is a parallelogram.

Important!

CPCT - Corresponding Parts of Congruence Triangles

Previous theory

Exit to the topic

Next theory

Send feedback

Did you find an error?

Send it to us!

2. Theorem I, II and III

Theory: