UPSKILL MATH PLUS
Learn Mathematics through our AI based learning portal with the support of our Academic Experts!
Learn moreTheorem VIII
A quadrilateral is a parallelogram if a pair of opposite sides is equal and parallel.
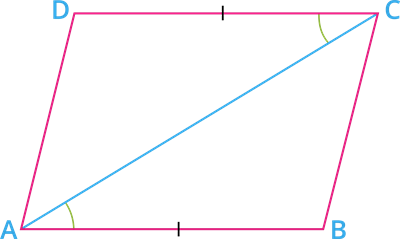
Given: \(ABCD\) is a quadrilateral, where \(AB = CD\) and \(AB \ || \ CD\).
To prove: \(ABCD\) is a parallelogram.
Proof: In \(\Delta ADC\) and \(\Delta CBA\):
\(AB = CD\) [Given]
\(\angle BAC = \angle ACD\) [Alternate interior angles]
\(CA = CA\) [Common side]
Therefore, \(\Delta ADC \cong \Delta CBA\) [by \(ASA\) congruence criterion].
\(\Rightarrow AD = BC\) [by CPCT] - - - - - (I)
We have, \(\angle BAC = \angle ACD\).
These are alternate angles.
The alternate interior angles theorem states that, "the alternate interior angles are congruent when the transversal intersects two parallel lines".
Here, \(AC\) is the transversal line for the lines \(AD\) and \(BC\).
So, \(AD \ || \ BC\) - - - - - (II)
From (I) and (II), we get:
\(AD \ || \ BC\) and \(AD = BC\)
Thus, both pair of opposite sides are equal and parallel.
Therefore, \(ABCD\) is a parallelogram.
Register for free to see more content