Properties of Matrix II — lesson. Mathematics State Board, Class 10.

PDF chapter test TRY NOW

Distributive property:

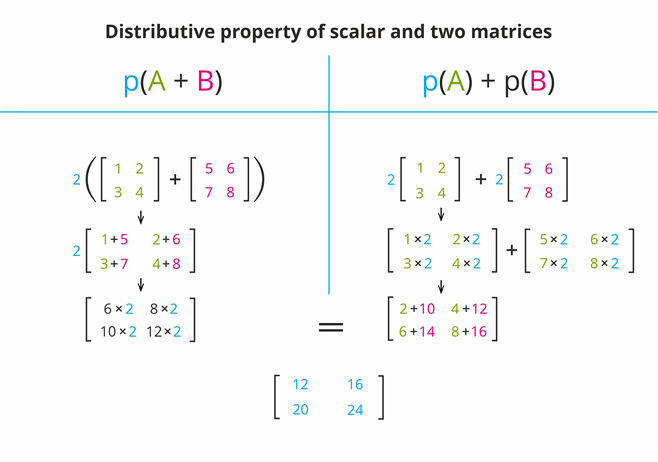
1. Distributive property of scalar and two matrices -

$p(A+B) = pA+ pB$

Let

$A$ , and

$B$ be

$m×n$ matrices and

$p$ and

$q$ be two non-zero scalars (numbers).

Example:

Consider the matrices

$A = \begin{bmatrix} 1 & 2 \\ 3 & 4 \end{bmatrix}, B = \begin{bmatrix} 5 & 6\\ 7 & 8 \end{bmatrix}$ and

$p = 2$ then verify

$p(A+B) = pA+ pB$ .

Solution:

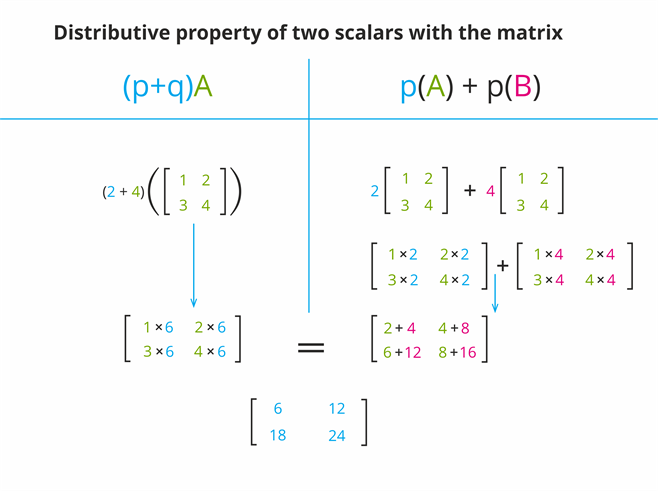
2. Distributive property of two scalars with a matrix -

$(p +q)A = pA +qA$

Example:

Let's take the same matrices to prove

$A = \begin{bmatrix} 1 & 2 \\ 3 & 4 \end{bmatrix}$ to prove

$(p +q)A = pA +qA$ . Where

$p = 2$ , and

$q = 4$ .

Solution:

Did you find an error?

4. Properties of Matrix II