PUMPA - SMART LEARNING
எங்கள் ஆசிரியர்களுடன் 1-ஆன்-1 ஆலோசனை நேரத்தைப் பெறுங்கள். டாப்பர் ஆவதற்கு நாங்கள் பயிற்சி அளிப்போம்
Book Free DemoLet us recall the definition of collinearity.
Three or more points are said to be collinear if they lie on the same line.
Let us learn how to find the collinearity of three points using the area of the triangle formula.
Consider the points \((3, 14)\), \((6, 11)\) and \((8, 9)\).
Let \((x_1, y_1)\) \(=\) \((3, 14)\), \((x_2, y_2)\) \(=\) \((6, 11)\) and \((x_3, y_3)\) \(=\)\((8, 9)\).
Now let us find the area of the triangle joining the given points.
We know that:
The area of the triangle having the vertices \((x_1, y_1)\), \((x_2, y_2)\) and \((x_3, y_3)\) is given by:
Area of the triangle \(=\) \(\frac{1}{2}\left[x_1 \left(y_2 - y_3\right) + x_2 \left(y_3 - y_1\right) + x_3 \left(y_1 - y_2\right)\right]\)
Substitute the required values in the above formula.
Area of the triangle \(=\) \(\frac{1}{2}\left[3 \left(11 - 9\right) + 6 \left(9 - 14\right) + 8 \left(14 - 11\right)\right]\)
\(=\) \(\frac{1}{2}\left[3 \left(2\right) + 6 \left(-5\right) + 8 \left(3\right)\right]\)
\(=\) \(\frac{1}{2}\left[(6) + (-30) + (24)\right]\)
\(=\) \(\frac{0}{2}\)
\(=\) \(0\)
Here, the area of the triangle joining the given points is \(0\) square unit.
It is impossible to have a triangle with \(0\) square unit area.
Let us plot the same points on the graph and find out the relation.
 |
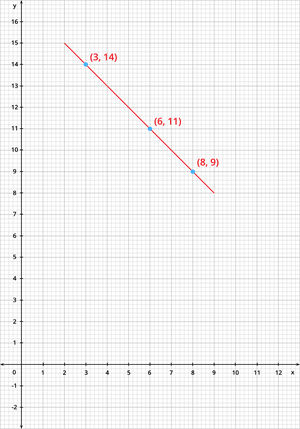From the graph, it is observed that the given points do not form a triangle. Instead, they are the points on the same line.
By the definition of collinearity, it is called collinear points.
Now let us re-define the definition of collinearity of three points using the area of the triangle as follows:
If the area of the triangle joining the points \((x_1, y_1)\), \((x_2, y_2)\) and \((x_3, y_3)\) is zero, then the points are said to be collinear.
Mathematically, \(\frac{1}{2}\left[x_1 \left(y_2 - y_3\right) + x_2 \left(y_3 - y_1\right) + x_3 \left(y_1 - y_2\right)\right]\) \(=\) \(0\).
Important!
Another condition for collinearity:
If the points \((x_1, y_1)\), \((x_2, y_2)\) and \((x_3, y_3)\) are collinear, then:
- \(x_1 \left(y_2 - y_3\right) + x_2 \left(y_3 - y_1\right) + x_3 \left(y_1 - y_2\right)\) \(=\) \(0\) (or)
- \(x_1y_2 + x_2y_3 + x_3y_1\) \(=\) \(x_2y_1 + x_3y_2 + x_1y_3\)
Register for free to see more content