UPSKILL MATH PLUS
Learn Mathematics through our AI based learning portal with the support of our Academic Experts!
Learn moreResult \(5\):
If two circles touch internally, the distance between their centres equals the difference in their radii.
Explanation:
 |
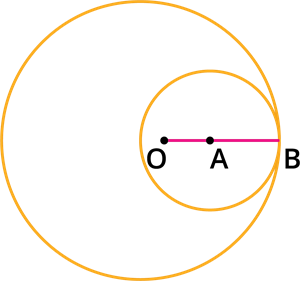If two circles touch internally at a point \(B\), then the distance \(OA\) is equal to the difference between the radii \(OB\) and \(AB\).
\(\Rightarrow\) \(OA\) \(=\) \(OB\) \(-\) \(AB\)
Proof of the result:
Let the two circles with centres \(O\) and \(A\) intersect each other internally at point \(B\).
Let the radius \(OB\) \(=\) \(r_{1}\) and \(AB\) \(=\) \(r_{2}\) and \(r_{1}\) \(>\) \(r_{2}\).
Let the distance between the centres be \(d\).
\(\Rightarrow\) \(OA\) \(=\) \(d\)
From the figure, we observe that \(OA\) \(=\) \(OB\) \(-\) \(AB\).
\(\Rightarrow\) \(d\) \(=\) \(r_{1}\) \(-\) \(r_{2}\)
Example:
Two circle with radii \(4\) \(cm\) and \(5\) \(cm\) intersect at a point \(O\) internally. If so, find the distance between their centres.
Solution:
By the result, we know that:
Distance between the centres \(=\) Difference of the radii.
Thus, the distance between the centres \(=\) \(5\) \(cm\) \(-\) \(4\) \(cm\)
\(=\) \(1\) \(cm\)
Register for free to see more content