PDF chapter test TRY NOW
Let \(A\) and \(B\) are the events of a random experiment.
Let \(S\) be the sample space where \(A \subseteq S\) and \(B \subseteq S\).
Based on the occurrence of the events \(A\) and \(B\), we shall discuss certain algebraic property.
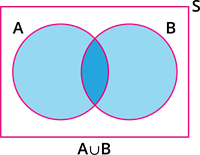
- The event \((A \cup B)\):
The events \((A \cup B)\) occurs only when at least one of the events \(A\) or \(B\) occurs.
This event is depicted using the Venn diagram as follows:
- The event \((A \cap B)\):
The events \((A \cap B)\) occurs only when both the events \(A\) and \(B\) occurs.
This event is depicted using the Venn diagram as follows:
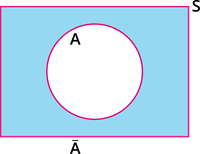
- The event \(\overline A\):
The event \(\overline A\) occurs only when the event \(A\) does not occurs.
This event is depicted using the Venn diagram as follows:
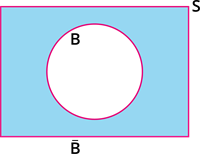
- The event \(\overline B\):
The event \(\overline B\) occurs only when the event \(B\) does not occurs.
This event is depicted using the Venn diagram as follows:
Important!
- \(P(A \cup \overline A) = S\)
- \(P(A \cap \overline A) = \phi\)
- \(\overline {A \cup B} = \overline A \cap \overline B\) represents the event when neither \(A\) nor \(B\) happens.
- If the events \(A\) and \(B\) are mutually exclusive, then \(P(A \cup B) = P(A) + P(B)\).
- \(P(\text{Union of events}) = \sum(\text{ Probability of events})\)
Register for free to see more content