2. Problems involving angle of elevation

The altitude of the plane from point \(C\) on the ground is \(1500 \ m\). If the angle of elevation of the person from point \(A\) is \(60^{\circ}\), then find the distance of the person from the point \(A\) to \(C\).

 

Solution:

 

 

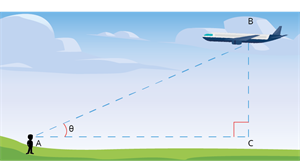
Let \(A\) be the position of the person, \(B\) be the position of the airplane, and \(C\) be the point.

 

Then, from the given data, we have:

 

\(\theta = 60^{\circ}\) and \(BC = 1500 \ m\)

 

In the right triangle \(ACB\), we have:

 

\(tan \ \theta = \frac{BC}{AC}\)

 

\(tan \ 60^{\circ} = \frac{1500}{AC}\)

 

\(AC = \frac{1500}{tan \ 60^{\circ}}\)

 

\(AC = \frac{1500}{\sqrt{3}}\)

 

\(AC = 500\sqrt{3}\)

 

Therefore, the distance between points \(A\) and \(C\) is \(500\sqrt{3} \ m\).