Problems involving angle of depression — lesson. Mathematics State Board, Class 10.

UPSKILL MATH PLUS

Learn Mathematics through our AI based learning portal with the support of our Academic Experts!

Learn more

Let us learn how to solve real-life situations based on the angle of depression.

Example:

1. A man observes the ball, which is at a distance of

$1.5 \ m$ from him. If the angle of depression is

$45^{\circ}$ , then find the height of the man.

Solution:

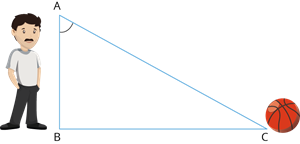
Let

$AB$ denote the height of the man and

$BC$ denote the distance of the man from the ball.

From the given data, we have:

$\theta = 45^{\circ}$ , and

$BC = 1.5 \ m$

To find: The height of the man (

$AB$ ).

Explanation:

In the right-angled

$\triangle ABC$ ,

$tan \ \theta = \frac{BC}{AB}$

$tan \ 45^{\circ} = \frac{1.5}{AB}$

$1 = \frac{1.5}{AB}$

$AB = 1.5 \ m$

Therefore, the height of the man is

$1.5 \ m$ .

2. A man observes a ball that is at a distance of

$1.2 \sqrt{3} \ m$ from him. If the height of the man is

$1.2 \ m$ , then find the angle of depression.

Solution:

Let

$AB$ denote the height of the man and

$BC$ denote the distance of the man from the ball.

From the given data, we have:

$AB = 1.2 \ m$ , and

$BC = 1.2 \sqrt{3} \ m$

In the right-angled triangle

$ABC$ ,

$tan \ \theta = \frac{BC}{AB}$

$tan \ \theta = \frac{1.2 \sqrt{3}}{1.2}$

$tan \ \theta = \sqrt{3}$

$\theta = 60^{\circ}$

Therefore, the angle of depression is

$60^{\circ}$ .

Important!

The angle of elevation and angle of depression are equal because they are alternate angles.

Previous theory

Exit to the topic

Next theory

Send feedback

Did you find an error?

Send it to us!

3. Problems involving angle of depression

Theory: