UPSKILL MATH PLUS
Learn Mathematics through our AI based learning portal with the support of our Academic Experts!
Learn moreRotational transformation:
An object rotating itself over a fixed point is called rotational transformation.
The fixed point is the centre of rotation.
The angle with which the object rotates is the angle of rotation.
The object always rotates in the anti-clockwise direction.
Example:
 | |
 |  |
 |  |
Let us analyze the example in detail.
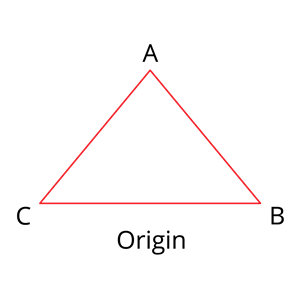
1. Origin is the original position of the figure.
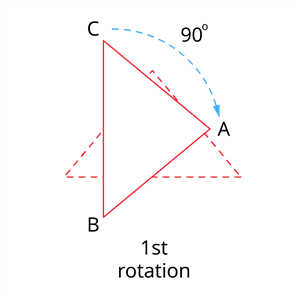
2. During the \({1^{st}}\) rotation the figure is rotated by a \(90°\).
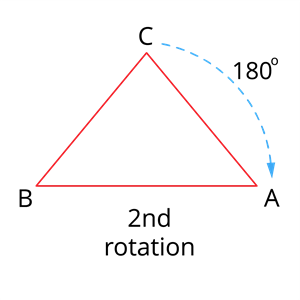
3. During the \({2^{nd}}\) rotation, the figure is rotated by another \(90°\).
\(=\) \(90°\) \(+\) \(90°\)
\(=\) \(180°\)
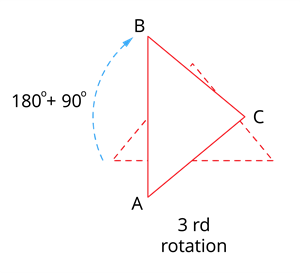
4. During the \({3^{rd}}\) rotation, the figure is rotated by another \(90°\).
\(=\) \(180°\) \(+\) \(90°\)
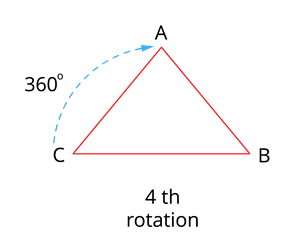
5. During the \({4^{th}}\) rotation, the figure is rotated by another \(90°\).
\(=\) \(180°\) \(+\) \(90°\) \(+\) \(90°\)
\(=\) \(360°\)
During the \({4^{th}}\) and the final rotation, the figure gets back to its original position.
Register for free to see more content