PUMPA - SMART LEARNING
எங்கள் ஆசிரியர்களுடன் 1-ஆன்-1 ஆலோசனை நேரத்தைப் பெறுங்கள். டாப்பர் ஆவதற்கு நாங்கள் பயிற்சி அளிப்போம்
Book Free DemoWe have gone through the fundamental idea of the Fibonacci sequence. Now let us explore how we can see it in nature.
Example:
Let us take the family tree of bees.
Before that, let's brush up with some basic biology.
- A male bee has one parent -----> Female bee.
- A female bee has two parents ------> Male and female bee.
[Note: The queen’s unfertilized eggs produce male bees, so male bees only have a mother but no father!]
Using this information now, we can draw the family tree for the male bee..
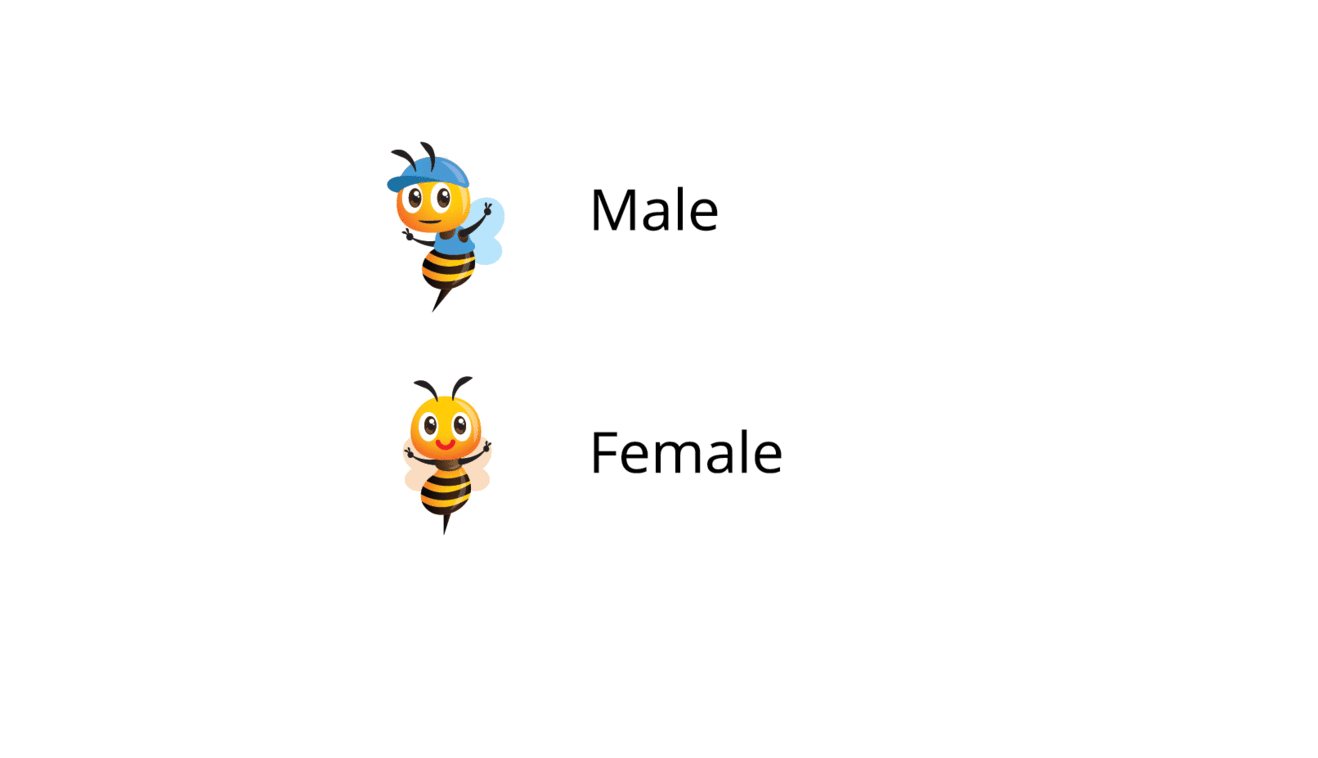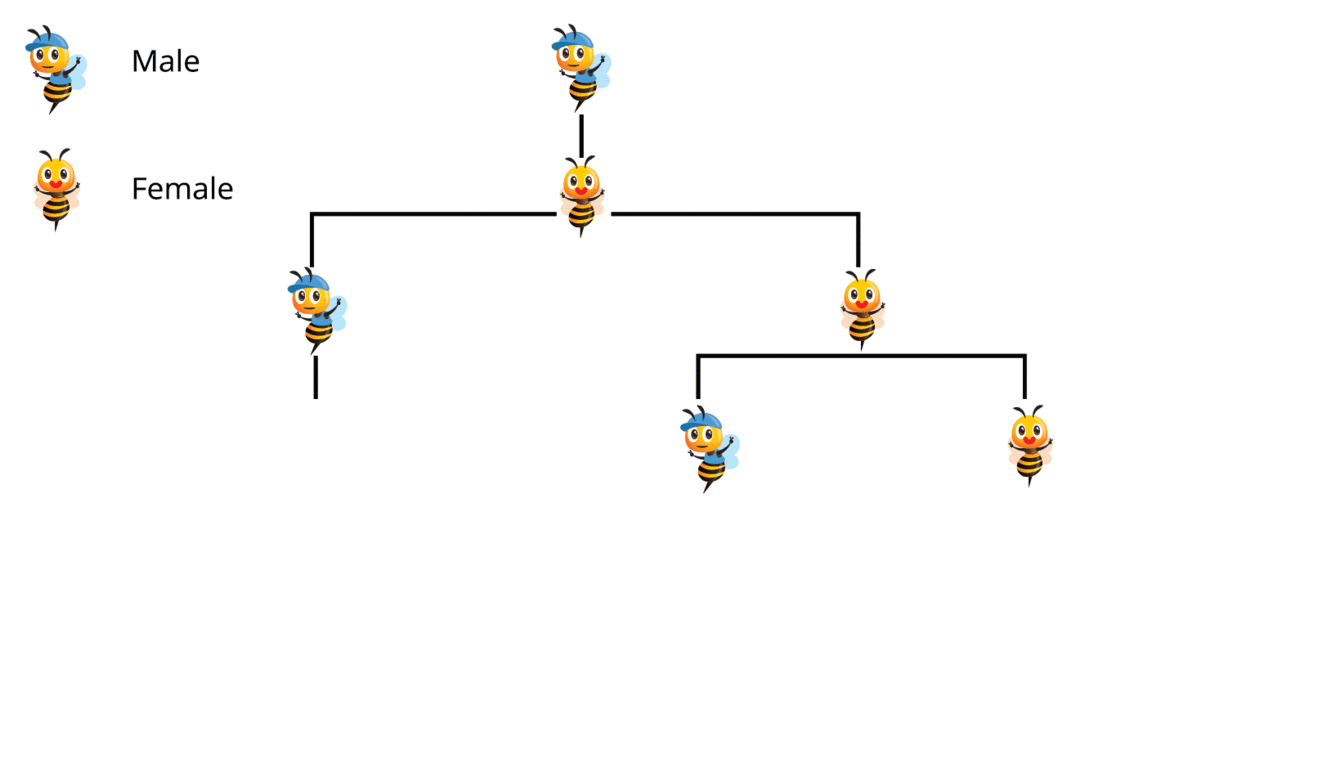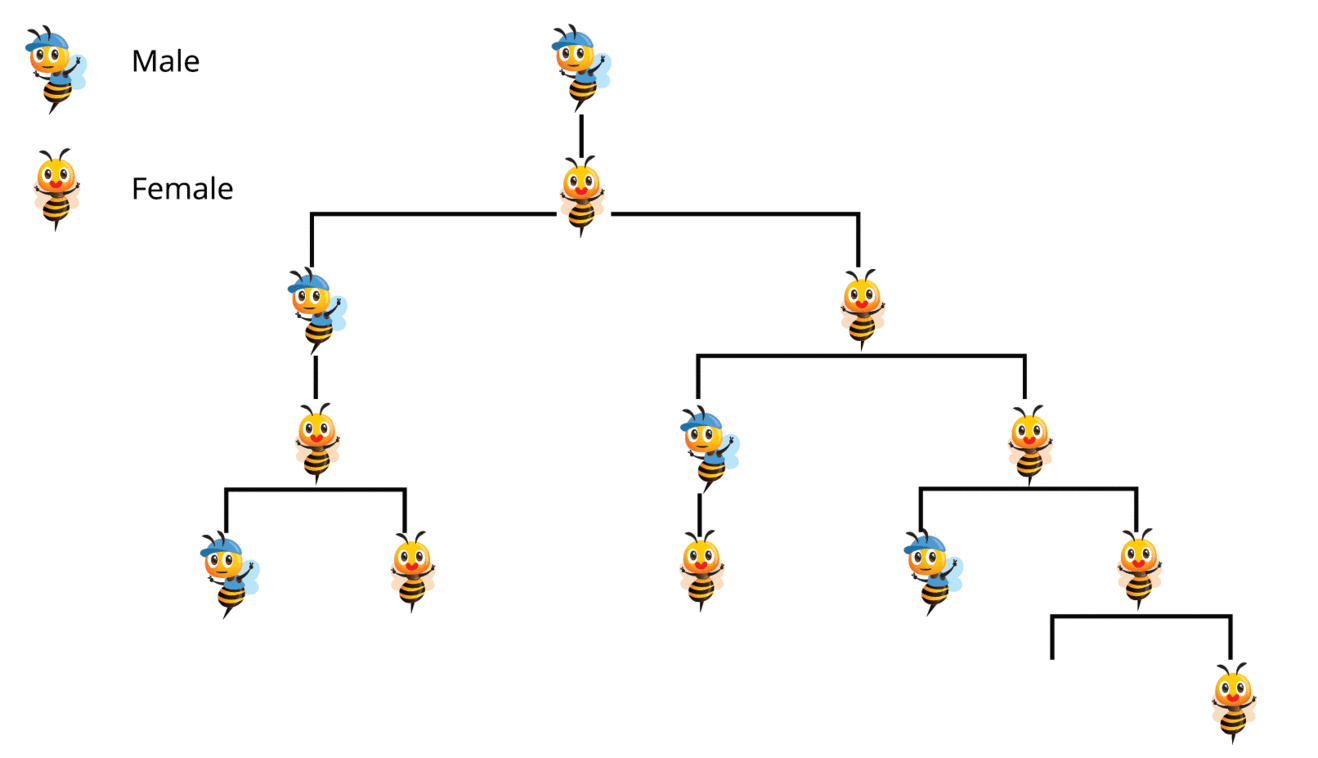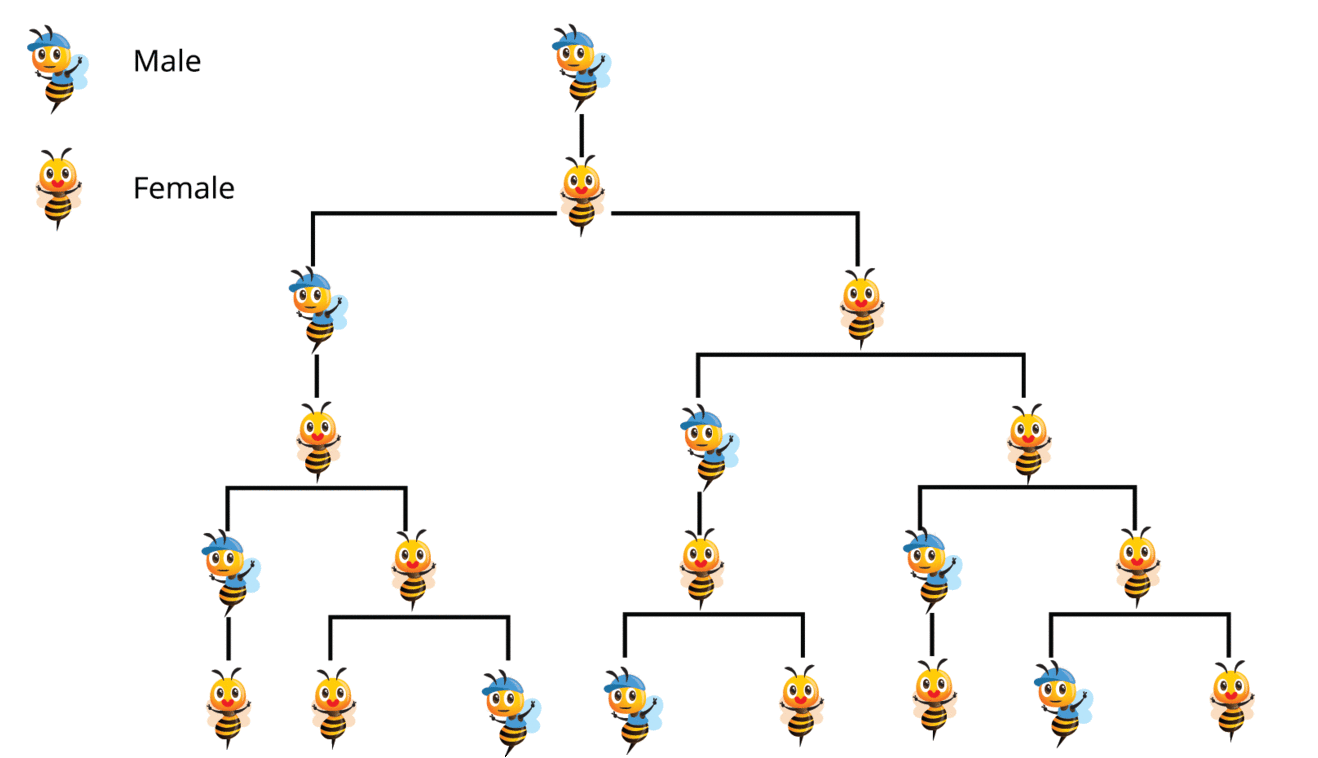
If we count how many bees are there in each layer, we get the count as shown below.
Yes! you read that right. Pattern of numbers \(1\), \(1\), \(2\), \(3\), \(5\), \(8\) is a Fibonacci sequence.
Bee population are not the only place in nature where the Fibonacci sequence occurs; they also appear in the beautiful shapes of trees, leaves, seeds, shells, storms.
If you visit your nearby park or plant gallery, we can probably see the Fibonacci sequence in the flowers' petals.
Now we see some flowers which have petals in the form of the Fibonacci sequence.
Example:
The following flowers have:
i) \(3\) petals.
ii) \(5\) petals
iii) \(8\) petals
iv) \(13\) petals
Now we understand that the flowers' petals follow the Fibonacci sequence. In the upcoming theory, we learn the pattern of the Fibonacci sequences.
Reference:
Frangipani flower image: Flickr.com - Swaminathan
https://www.flickr.com/photos/araswami/2630033130
Register for free to see more content