PDF chapter test TRY NOW
Illustration:
A four-sided figure inscribed in a circle such that all its vertices lie on the circumference of the circle is said to be a cyclic quadrilateral.
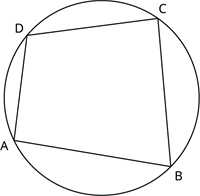
Consider a quadrilateral ABCD whose vertices lie on the circumference of the circle with centre O.
Connect all the four vertices with centre O to get four isosceles triangle AOB, BOC, COD and DOA where the sides OA, OB, OC and OD are the radii.
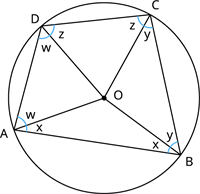
The sum of the angles around the centre of a circle is 360^{\circ}.
Also, the sum of all the interior angles of a triangle is 180^{\circ}.
Hence:
Adding all the equations we have:
2 (\angle w + \angle x + \angle y + \angle z) + \angle O = 4\times180^{\circ}
2 (\angle w + \angle x + \angle y + \angle z) + 360^{\circ} = 720^{\circ}
2 (\angle w + \angle x + \angle y + \angle z) = 720^{\circ} - 360^{\circ}
2 (\angle w + \angle x + \angle y + \angle z) = 360^{\circ}
\angle w + \angle x + \angle y + \angle z = 180^{\circ}
This is rewritten as, \angle A + \angle C = 180^{\circ}.
Similarly, \angle B + \angle D = 180^{\circ}.
Based on this result, the following theorem is obtained.
Theorem: Opposite angles of a cyclic quadrilateral are supplementary.
Explanation:

The theorem states that the sum of the interior opposite angles of a cyclic quadrilateral is 180^{\circ}.
That is, \angle A + \angle C = 180^{\circ}.
And \angle B + \angle D = 180^{\circ}.
Example:
Find the unknown angle x in the given figure.

Solution:
By the theorem, opposite angles of a cyclic quadrilateral are supplementary.
This implies:
\angle A + \angle C = 180^{\circ}
x + 115^{\circ} = 180^{\circ}
x = 180^{\circ} - 115^{\circ}
x = 65^{\circ}
Converse of Theorem: If a pair of opposite angles of a quadrilateral is supplementary, then the quadrilateral is cyclic.
Explanation:
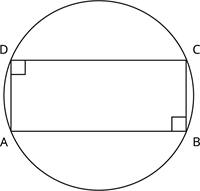
The theorem states that if the sum of the interior opposite angles of any quadrilateral is 180^{\circ}, then that quadrilateral is said to be cyclic. Here, in the figure, the sum of the \angle A and \angle C is 180^{\circ}.
90^{\circ} + 90^{\circ} = 180^{\circ}
Thus, the quadrilateral ABCD is cyclic.
Example:
Prove that a square inscribed in a circle is cyclic.
Proof:
Let ABCD be the square inscribed in a circle.

It is known that every angle of a square is 90^{\circ}.
Here \angle A + \angle C = 90^{\circ} + 90^{\circ}
Implies, \angle A + \angle C = 180^{\circ}
Similarly, \angle B + \angle D = 90^{\circ} + 90^{\circ}
Implies, \angle B + \angle D = 180^{\circ}
According to the theorem, the pair of opposite angles of the square is supplementary.
Hence the square inscribed in a circle is cyclic.
