PUMPA - SMART LEARNING
எங்கள் ஆசிரியர்களுடன் 1-ஆன்-1 ஆலோசனை நேரத்தைப் பெறுங்கள். டாப்பர் ஆவதற்கு நாங்கள் பயிற்சி அளிப்போம்
Book Free DemoIllustration:
Consider a cyclic quadrilateral \(ABCD\) and extend the side \(AB\) to \(E\).
Here, the angles \(\angle ABC\) and \(\angle CBE\) are a linear pair.
It is known that the sum of the linear angle is \(180^{\circ}\).
So, \(\angle ABC\) \(+\) \(\angle CBE\) \(=\) \(180^{\circ}\) \(……\) \(\text{equation }(1)\)
Also, in a cyclic quadrilateral, the sum of the interior opposite angles is \(180^{\circ}\).
So, \(\angle ABC\) \(+\) \(\angle ADC\) \(=\) \(180^{\circ}\) \(……\) \(\text{equation }(2)\)
From equation \((1)\) and \((2)\), we have:
\(\angle ABC\) \(+\) \(\angle CBE\) \(=\) \(\angle ABC\) \(+\) \(\angle ADC\)
Hence, \(\angle CBE\) \(=\) \(\angle ADC\).
Similarly, all the other angles can be proved.
Based on this result, the following theorem is obtained.
Theorem: If one side of a cyclic quadrilateral is produced, then the exterior angle is equal to the interior opposite angle.
Explanation:
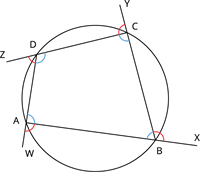
The theorem states that if any side of a cyclic quadrilateral is produced (extended), then the exterior angle formed on that side is the same as the interior opposite angle.
Here, in the figure, the side \(AB\) is produced to \(X\), the side \(BC\) is produced to \(Y\), the side \(CD\) is produced to \(Z\) and the side \(DA\) is produced to \(W\). Then:
\(\angle CBX = \angle CDA\)
\(\angle DCY = \angle DAB\)
\(\angle ADZ = \angle ABC\)
\(\angle BAW = \angle BCD\)
Example:
Find the unknown angle \(z\) in the figure.
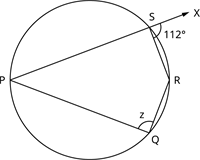
Solution:
By the exterior angle of a cyclic quadrilateral theorem, \(\text{Exterior angle}\) \(=\) \(\text{Interior opposite angle}\).
Hence, \(\angle RSX\) \(=\) \(\angle PQR\)
Therefore, \(z\) \(=\) \(112^{\circ}\).
Register for free to see more content