PUMPA - SMART LEARNING
எங்கள் ஆசிரியர்களுடன் 1-ஆன்-1 ஆலோசனை நேரத்தைப் பெறுங்கள். டாப்பர் ஆவதற்கு நாங்கள் பயிற்சி அளிப்போம்
Book Free DemoThis procedure was found before the calculator was not invented. It was tedious work to find the approximate value of the irrational number.
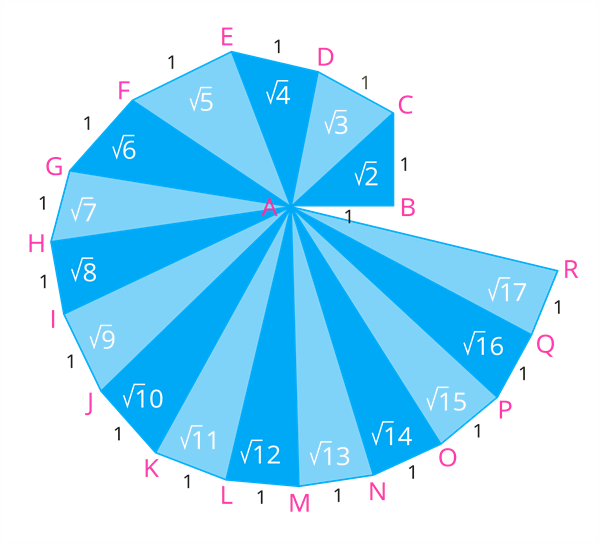
The ancient mathematicians use the fact that the square with side length \(1\) unit gives the diagonal length units. Keeping this diagonal value and drawing another side length with \(1\) unit results in units. Extending the unit side length, again and again, result in the square root of any number.
Thus, we have the following fact:
We can locate for any positive integer \(n\), after .
- Locate point A and draw a line segment AB of length \(1\) unit.
- Sketch a line segment BC of length \(1\) unit which is perpendicular to AB.
- Join AC. Now we have a right triangle ABC with AB = BC = \(1\) unit. By Pythagoras theorem, AC must be .
- Sketch a line segment CD of length \(1\) unit which is perpendicular to AC .
- Repeat the process to create square root spiral made up of line segments AC, AD, AE, … etc.
- Note that, AC, AD, AE , … denote the lengths , ,, , , , , , , ,...
Register for free to see more content