PUMPA - SMART LEARNING
எங்கள் ஆசிரியர்களுடன் 1-ஆன்-1 ஆலோசனை நேரத்தைப் பெறுங்கள். டாப்பர் ஆவதற்கு நாங்கள் பயிற்சி அளிப்போம்
Book Free DemoBased on the three basic trigonometric ratios \sin, \cos and \tan we will define its reciprocals.
Reciprocal Ratios:
Consider a right-angled triangle with a corresponding angle \theta.
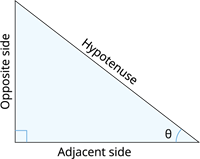
The three basic trigonometric ratios are:
- Sine
- Cosine
- Tangent
The table below depicts the relation of reciprocal ratios with the right-angled triangle.
| Name of the angle | Sine | Cosine | Tangent |
| Short form of the angle | \sin | \cos | \tan |
| Relationship | \sin \theta = \frac{\text{Opposite side}}{\text{Hypotenuse}} | \cos \theta = \frac{\text{Adjacent side}}{\text{Hypotenuse}} | \tan \theta = \frac{\text{Opposite side}}{\text{Adjacent side}} |
| Name of the reciprocal angle | Cosecant | Secant | Cotangent |
| Short form of the angle | \text{cosec} | \sec | \cot |
| Measurements related to the right-angled triangle |  |  |  |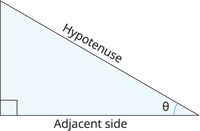
| Relationship | \text{cosec}\,\theta = \frac{\text{Hypotenuse}}{\text{Opposite side}} | \sec \theta = \frac{\text{Hypotenuse}}{\text{Adjacent side}} | \cot \theta = \frac{\text{Adjacent side}}{\text{Opposite side}} |
| Relation with the basic ratio | \text{cosec}\,\theta = \frac{1}{\sin \theta} or \sin \theta = \frac{1}{\text{cosec}\,\theta} | \sec \theta = \frac{1}{\cos \theta} or \cos \theta = \frac{1}{\sec \theta} | \cot \theta = \frac{1}{\tan \theta} or \tan \theta = \frac{1}{\cot \theta} |
We can write certain identities based on these relationships.
- Identity 1:
\text{cosec}\,\theta \times \sin \theta = \text{cosec}\,\theta \times \frac{1}{\text{cosec}\,\theta}
= 1
Therefore, \text{cosec}\,\theta \cdot \sin \theta= 1.
- Identity 2:
\sec \theta \times \cos \theta = \sec \theta \times \frac{1}{\sec \theta}
= 1
Therefore, \sec \theta \cdot \cos \theta = 1.
- Identity 3:
\cot \theta \times \tan \theta = \cot \theta \times \frac{1}{\cot \theta}
= 1
Therefore, \cot \theta \cdot \tan \theta = 1.
Register for free to see more content